Proposition I.35
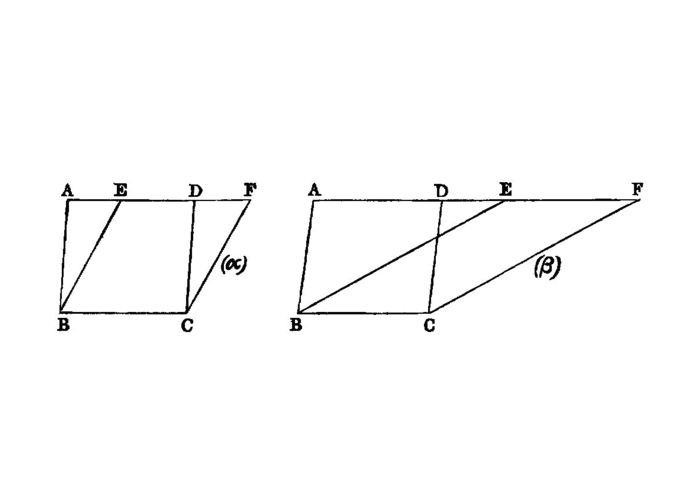
Parallelograms which are on the same base and in the same parallels are equal to one another.
Let ABCD, EBCF be parallelograms on the same base BC and in the same parallels AF, BC; I say that ABCD is equal to the parallelogram EBCF.
For, since ABCD is a parallelogram,
ADis equal toBC. I.34
For the same reason also
EFis equal toBC, so thatADis also equal toEF; I.c.n.1
and DE is common;
- therefore the whole
AEis equal to the wholeDF. I.c.n.2
But AB is also equal to DC; I.34 therefore the two sides EA, AB are equal to the two sides FD, DC respectively,
- and the angle
FDC1 is equal to the angleEAB, the exterior to the interior; I.29 therefore the baseEBis equal to the baseFC, and the triangleEABwill be equal to the triangleFDC. I.4
Let DGE be subtracted 2 from each; therefore the trapezium ABGD which remains is equal to the trapezium EGCF which remains. I.c.n.3
Let the triangle GBC be added to each; therefore the whole parallelogram ABCD is equal to the whole parallelogram EBCF. I.c.n.2
Therefore etc.
- Q. E. D.
