Mission
establish a framework for the study and codification of Euclid's Elements
Recast Euclid's Elements into a state-of-the-art framework
Recast Euclid's Elements into a state-of-the-art framework
Based on the works of Thomas Little Heath
This digital reference of Euclid's Elements is assembled from several English translations from over 100 years ago that are now in the public domain. The purpose of this project is to facilitate research and study of the dense information of the 13 books through intelligent navigation, robust taxonomies and comprehensive search.
Theory of angles, triangles, parallel lines, and parallelograms.
A
A
The
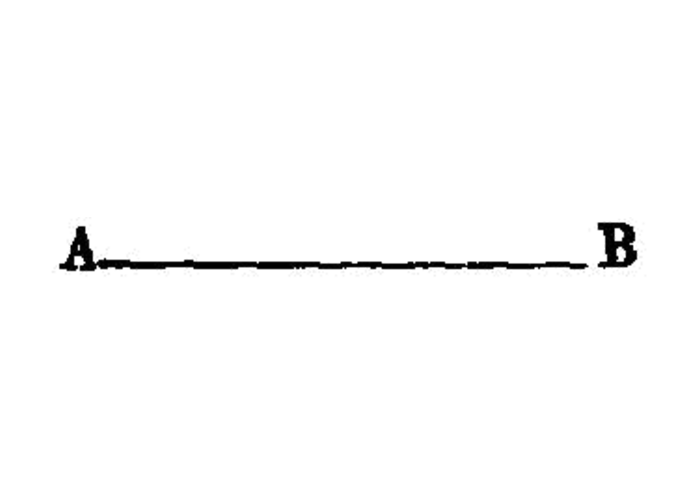
A
A
The
A
A
And when the lines containing the angle are straight, the angle is called
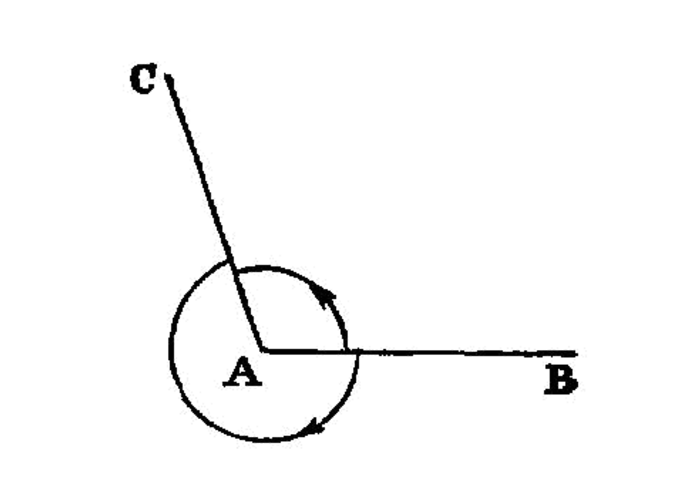
When a straight line set up on a straight line makes the adjacent angles equal to one another, each of the equal angles is
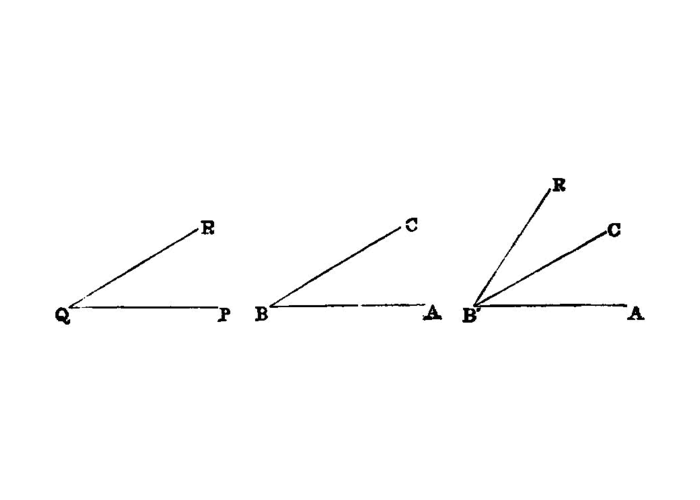
An
An
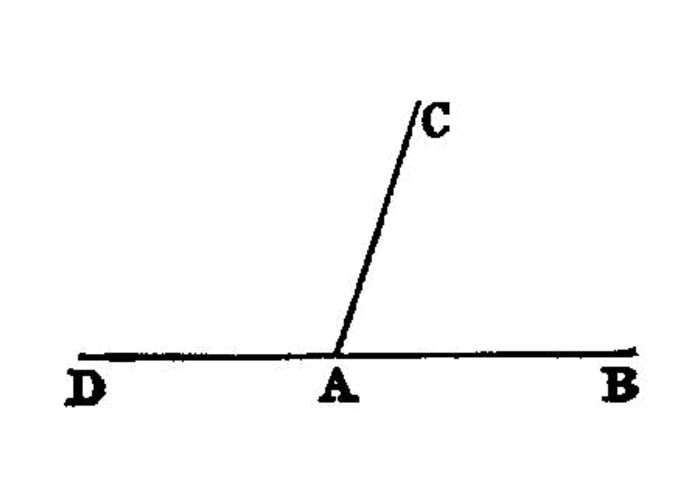
A
A
A
And the point is called the
A
A
Of
Further, of trilateral figures, a right-angled triangle is that which has a right angle, an obtuse-angled triangle that which has an obtuse angle, and an acute angled triangle that which has its three angles acute.
Of quadrilateral figures, a square is that which is both equilateral and right-angled; an oblong that which is right-angled but not equilateral; a rhombus that which is equilateral but not right-angled; and a rhomboid that which has its opposite sides and angles equal to one another but is neither equilateral nor right-angled. And let quadrilaterals other than these be called trapezia.
Parallel straight lines are straight lines which, being in the same plane and being produced indefinitely in both directions, do not meet one another in either direction.
To draw a straight line from any point to any point.
To produce a finite straight line continuously in a straight line.
To describe a circle with any centre and distance.
That all right angles are equal to one another.
That, if a straight line falling on two straight lines make the interior angles on the same side less than two right angles, the two straight lines, if produced indefinitely, meet on that side on which are the angles less than the two right angles.
Things which are equal to the same thing are also equal to one another.
If equals be added to equals, the wholes are equal.
If equals be subtracted from equals, the remainders are equal.
Things which coincide with one another are equal to one another.
The whole is greater than the part.

On a given finite straight line 1 to construct an equilateral triangle.
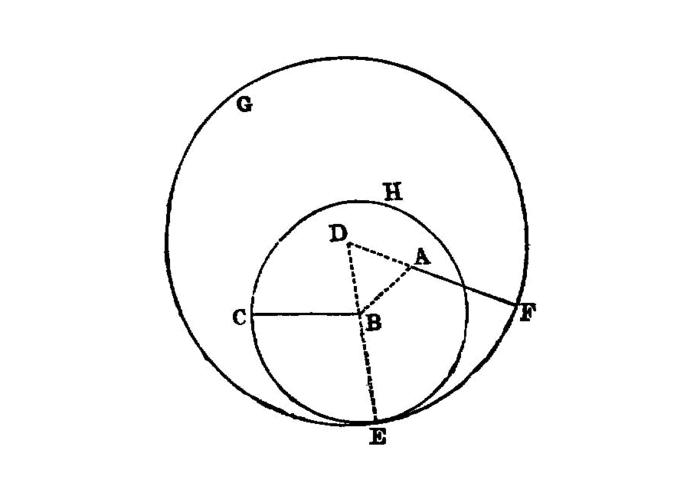
To place at a given point (as an extremity) a straight line equal to a given straight line.

Given two unequal straight lines, to cut off from the greater a straight line equal to the less.
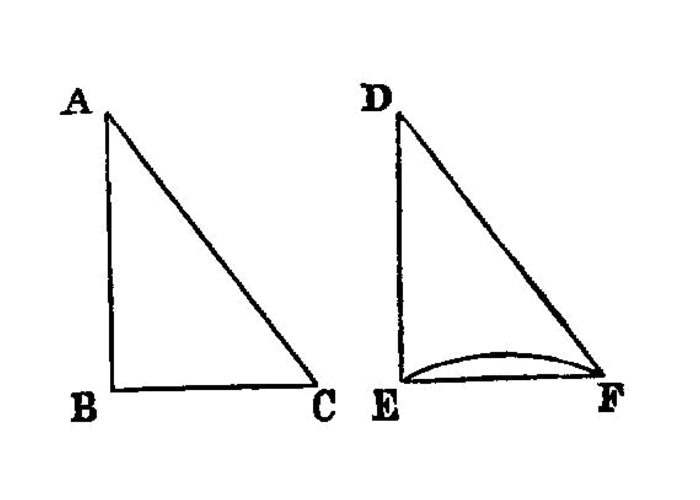
If two triangles have the two sides equal to two sides respectively, and have the angles contained by the equal straight lines equal, they will also have the base 1 equal to the base, the triangle will be equal to the triangle, and the remaining angles 2 will be equal to the remaining angles respectively, 3 namely those which the equal sides subtend. 4
In isosceles triangles the angles at the base are equal to one another, and, if the equal straight 1 lines be produced further, the angles under the base will be equal to one another.
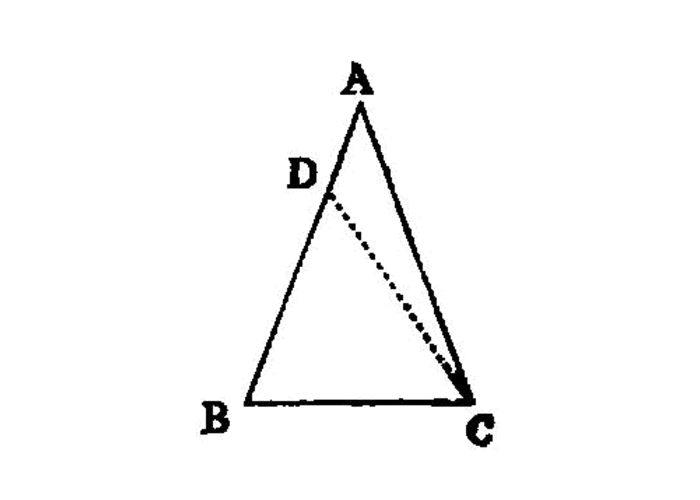
If in a triangle two angles be equal to one another, the sides which subtend the equal angles will also be equal to one another.

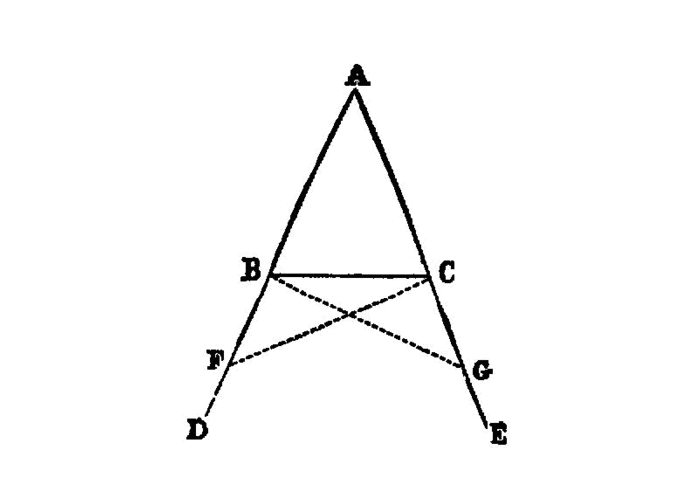
Given two straight lines constructed on a straight line (from its extremities) and meeting in a point, there cannot be constructed on the same straight line (from its extremities), and on the same side of it, two other straight lines meeting in another point and equal to the former two respectively, namely each to that which has the same extremity with it.
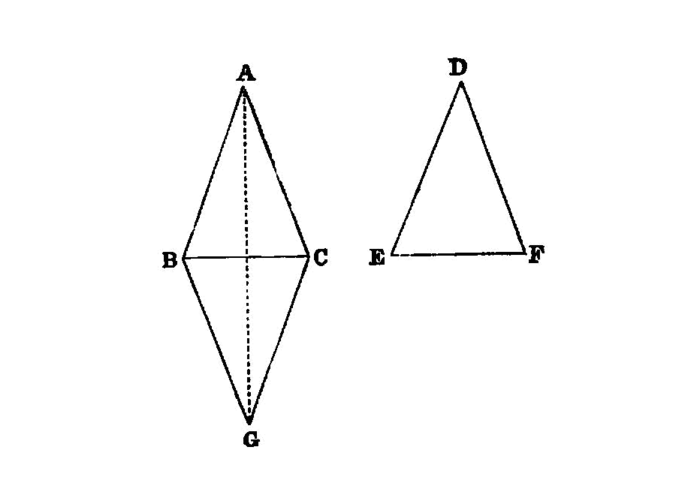
If two triangles have the two sides equal to two sides respectively, and have also the base equal to the base, they will also have the angles equal which are contained by the equal straight lines.

To bisect a given rectilineal angle.
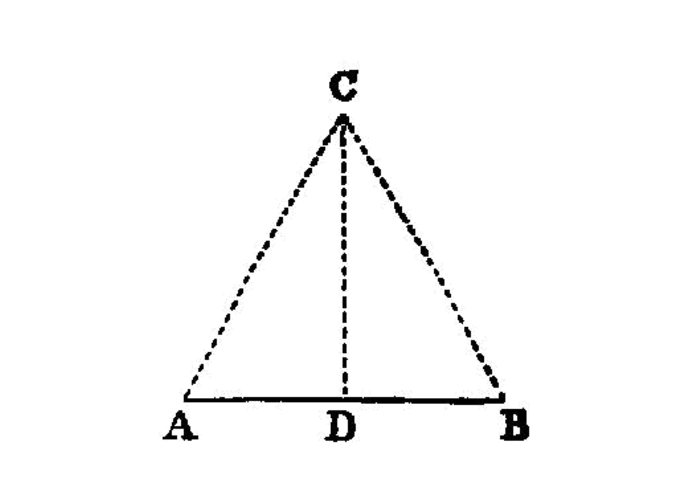
To bisect a given finite straight line.
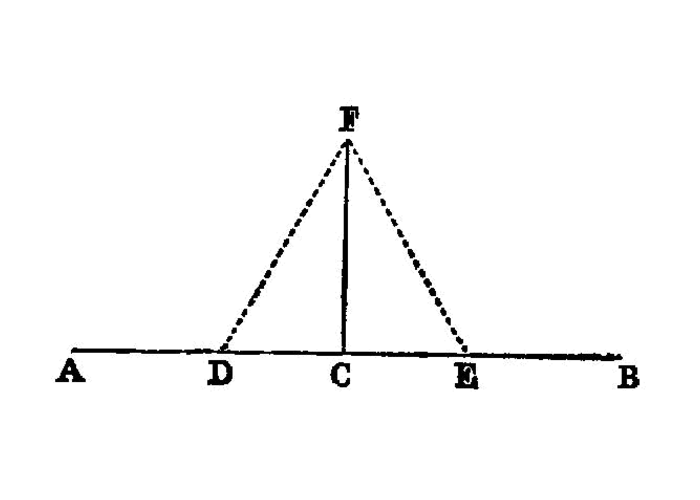
To draw a straight line at right angles to a given straight line from a given point on it.
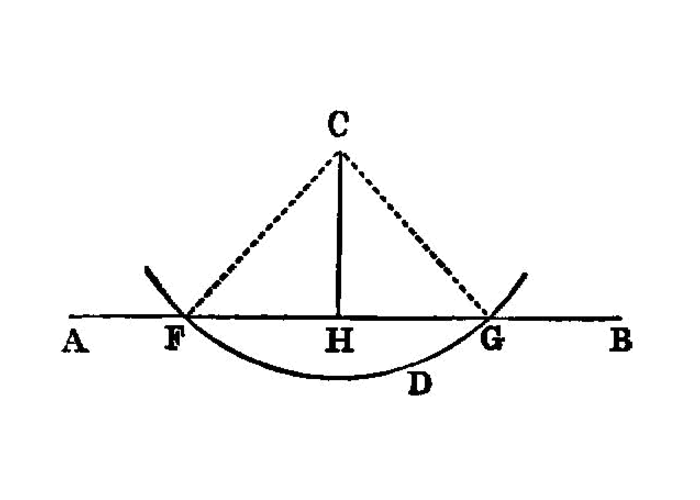
To a given infinite straight line, from a given point which is not on it, to draw a perpendicular straight line.1
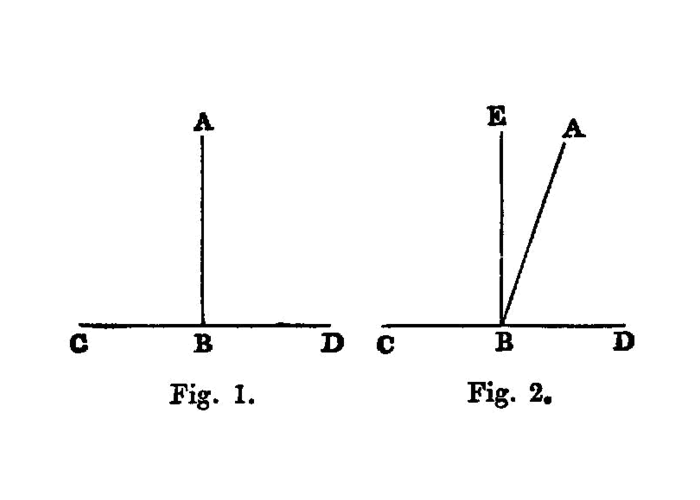
If a straight line set up on a straight line make angles, it will make either two right angles or angles equal to two right angles.

If with any straight line, and at a point on it, two straight lines not lying on the same side make the adjacent angles equal to two right angles, the two straight lines will be in a straight line with one another. 1
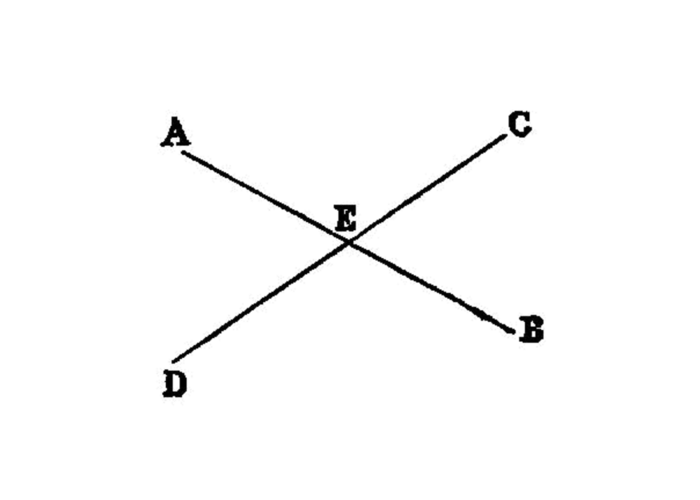
If two straight lines cut one another, they make the vertical angles 1 equal to one another.
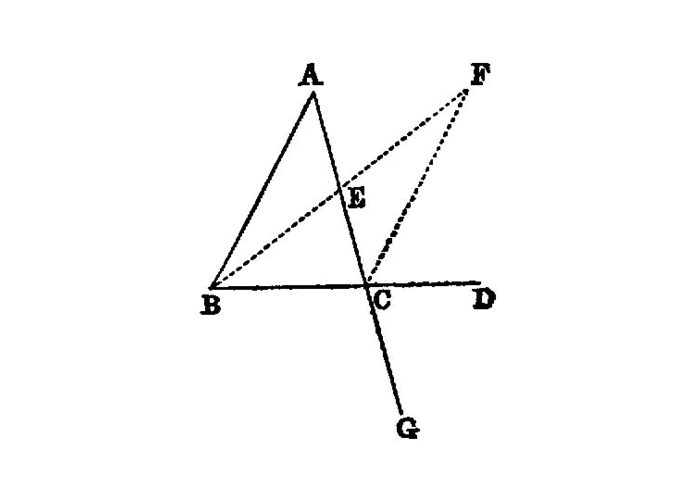
In any triangle, if one of the sides be produced, the exterior angle is greater than either of the interior and opposite angles.
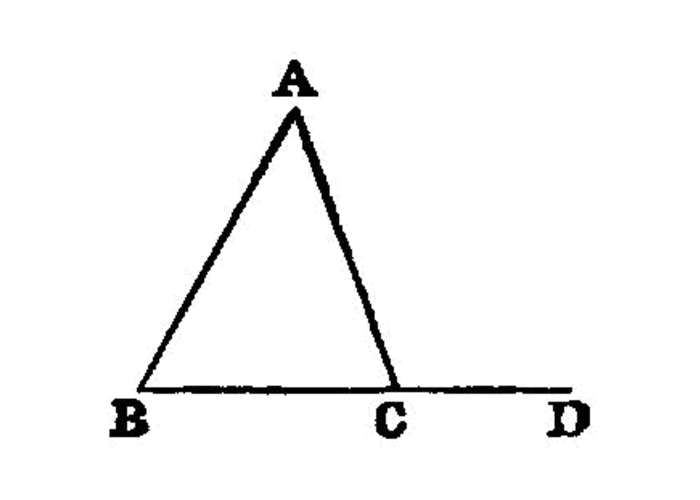
In any triangle two angles taken together in any manner 1 are less than two right angles.

In any triangle the greater side subtends the greater angle.
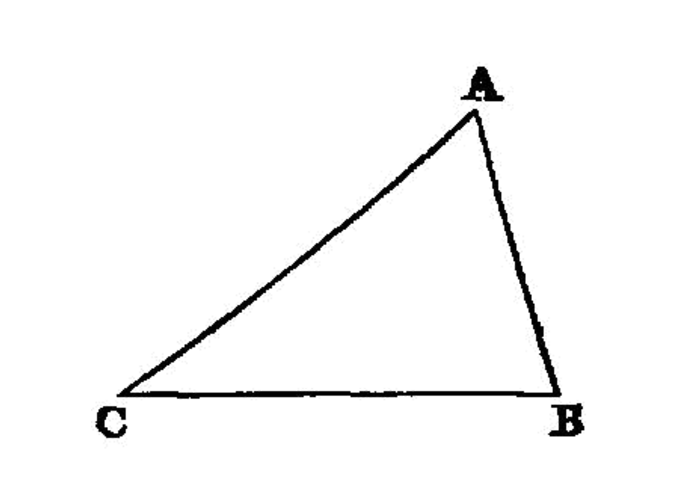
In any triangle the greater angle is subtended by the greater side.
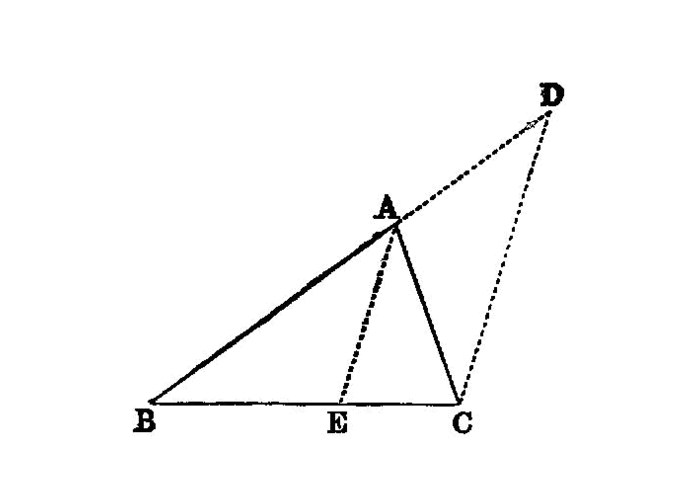
In any triangle two sides taken together in any manner are greater than the remaining one. 1
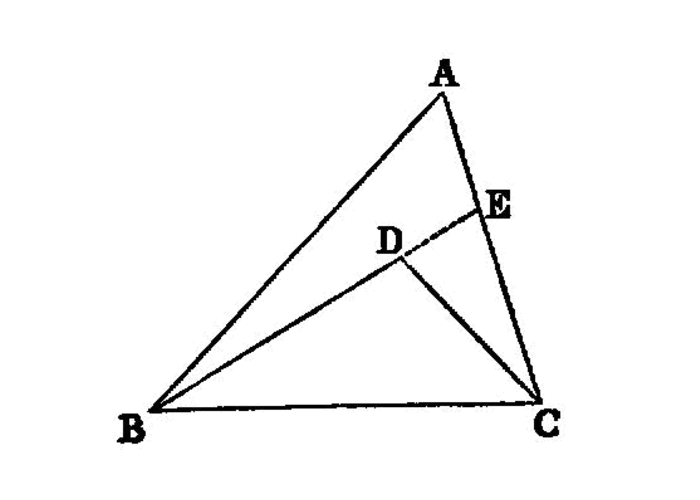
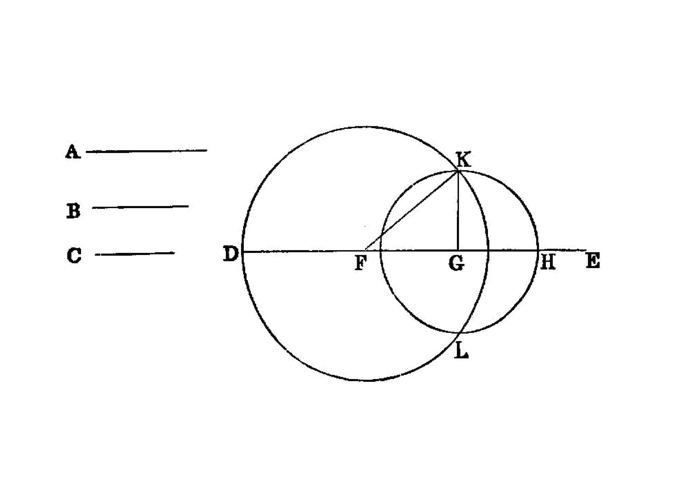
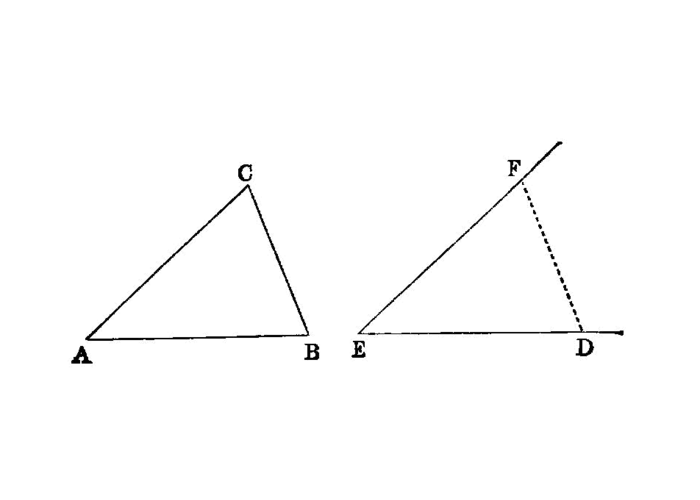
On a given straight line and at a point on it to construct a rectilineal angle equal to a given rectilineal angle.
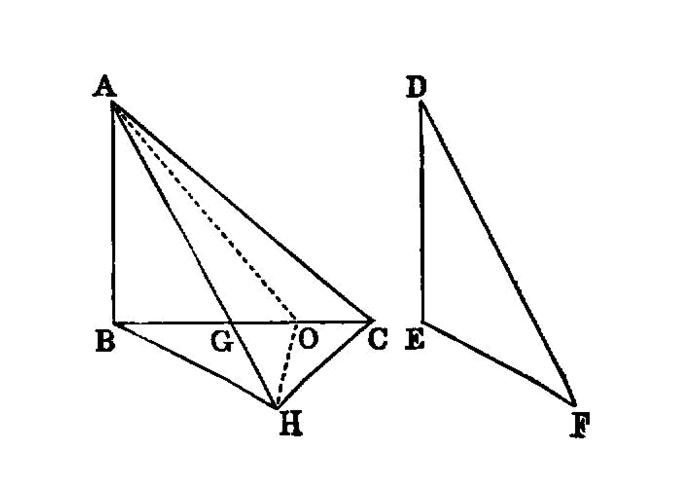
If two triangles have the two sides equal to two sides respectively, but have the one of the angles contained by the equal straight lines greater than the other, they will also have the base greater than the base.
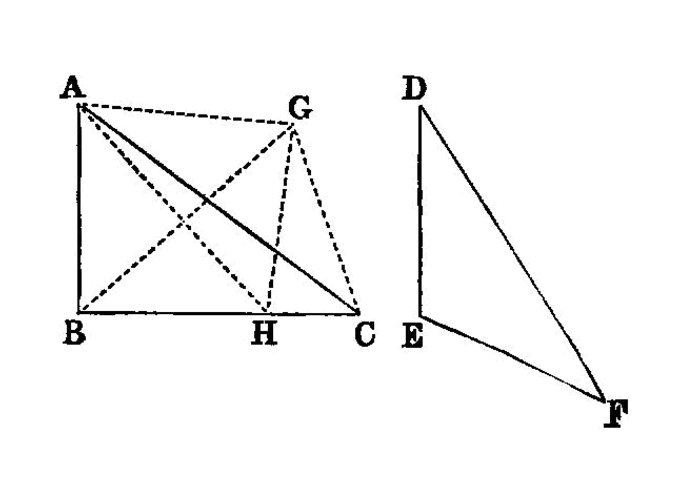
If two triangles have the two sides equal to two sides respectively, but have the base greater than the base, they will also have the one of the angles contained by the equal straight lines greater than the other.
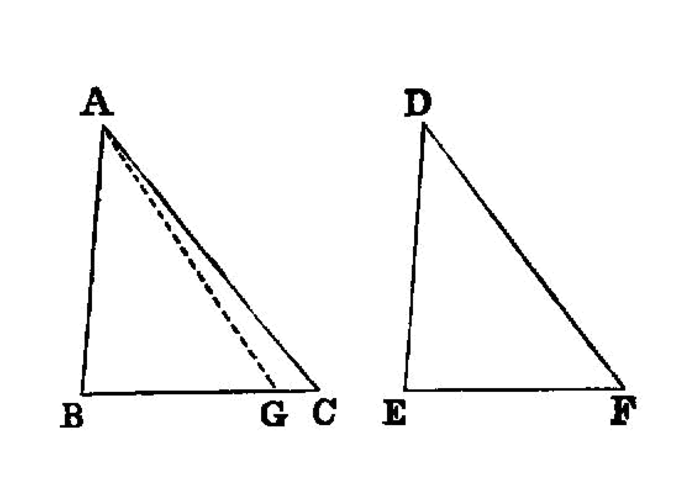
If two triangles have the two angles equal to two angles respectively, and one side equal to one side, namely, either the side adjoining the equal angles, 1 or that subtending one of the equal angles, they will also have the remaining sides equal to the remaining sides and the remaining angle to the remaining angle.
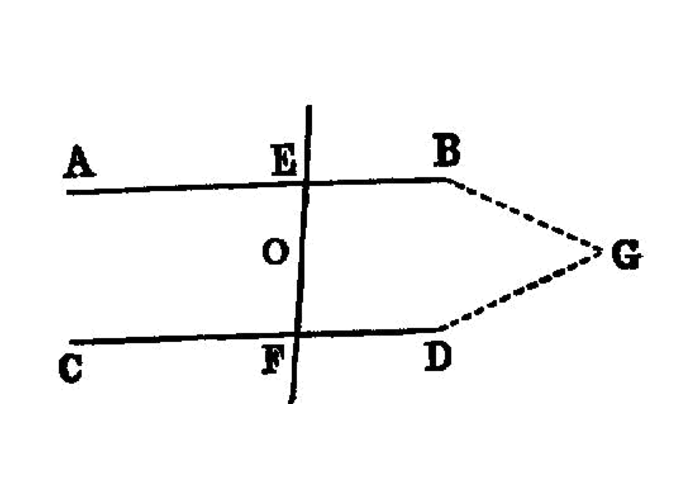
If a straight line falling on two straight lines 1 make the alternate angles [^I.27:2] equal to one another, the straight lines will be parallel to one another.
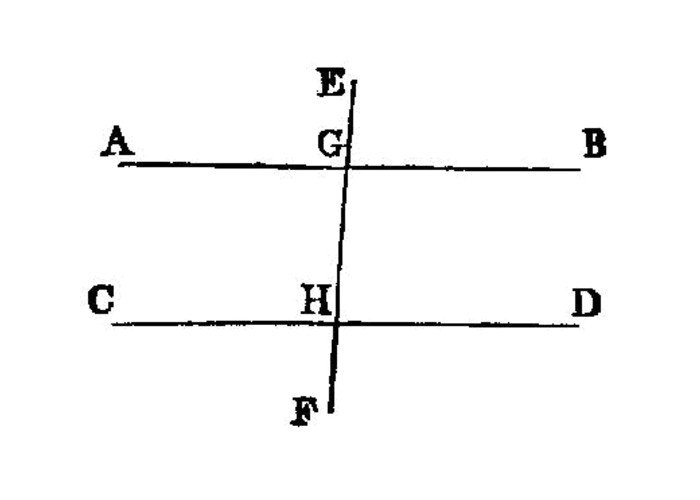
If a straight line falling on two straight lines make the exterior angle equal to the interior and opposite angle on the same side, or the interior angles on the same side equal to two right angles, the straight lines will be parallel to one another.
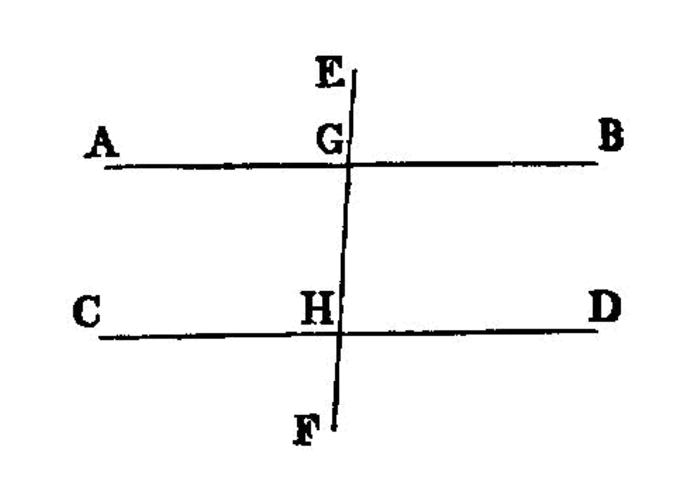
A straight line falling on parallel straight lines makes the alternate angles equal to one another, the exterior angle equal to the interior and opposite angle, and the interior angles on the same side equal to two right angles.
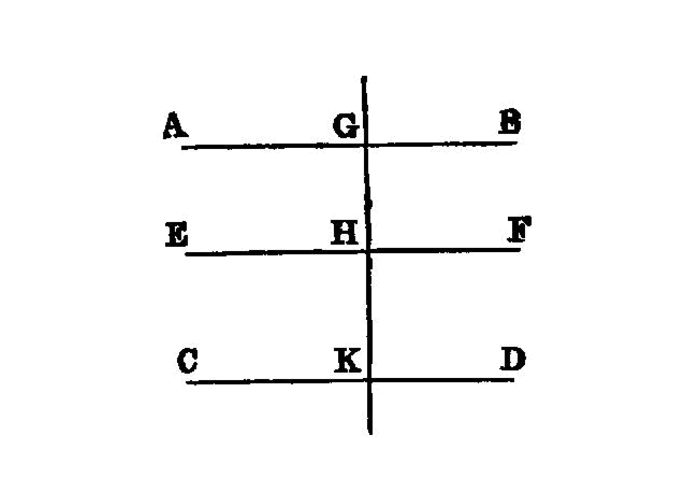
Straight lines parallel to the same straight line are also parallel to one another.

Through a given point to draw a straight line parallel to a given straight line.
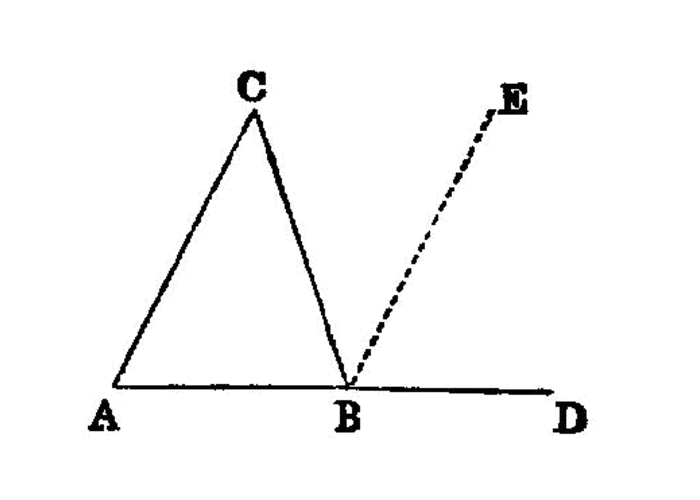
In any triangle, if one of the sides be produced, the exterior angle is equal to the two interior and opposite angles, and the three interior angles of the triangle are equal to two right angles.
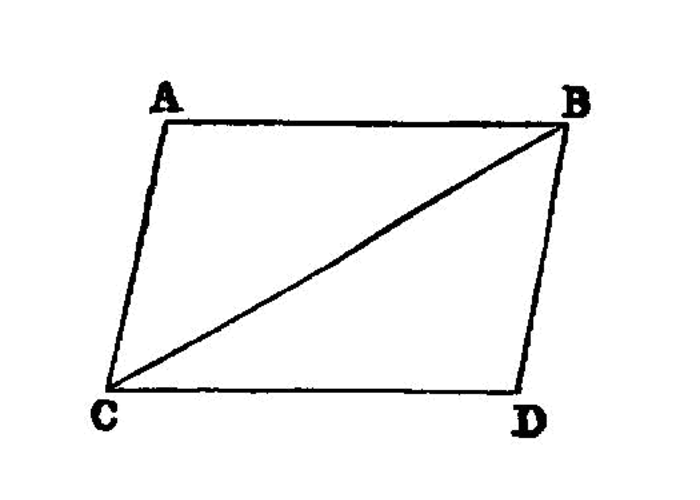
The straight lines joining equal and parallel straight lines (at the extremities which are) in the same directions (respectively) [^I.33:1] are themselves also equal and parallel.
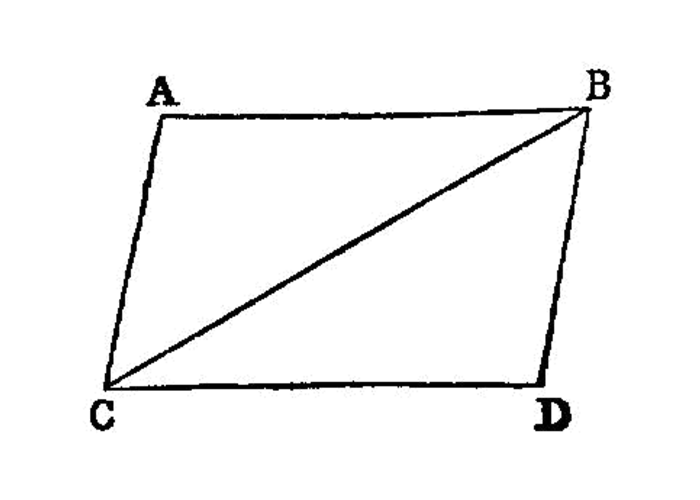
In parallelogrammic areasi 1 the opposite sides and angles are equal to one another, and the diameter bisects the areas.
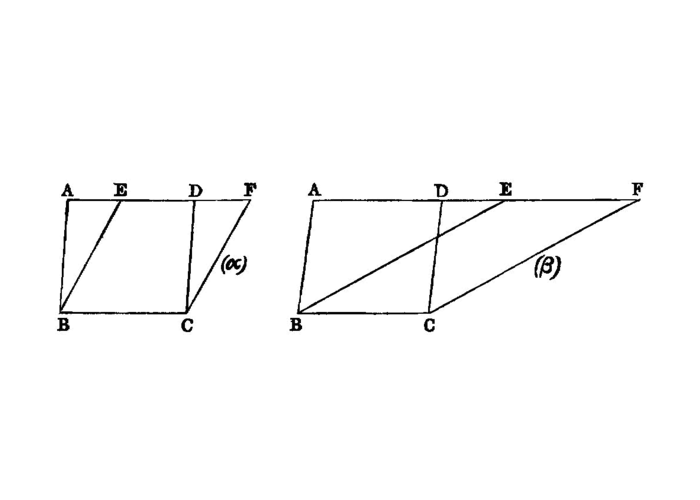
Parallelograms which are on the same base and in the same parallels are equal to one another.

Parallelograms which are on equal bases and in the same parallels are equal to one another.
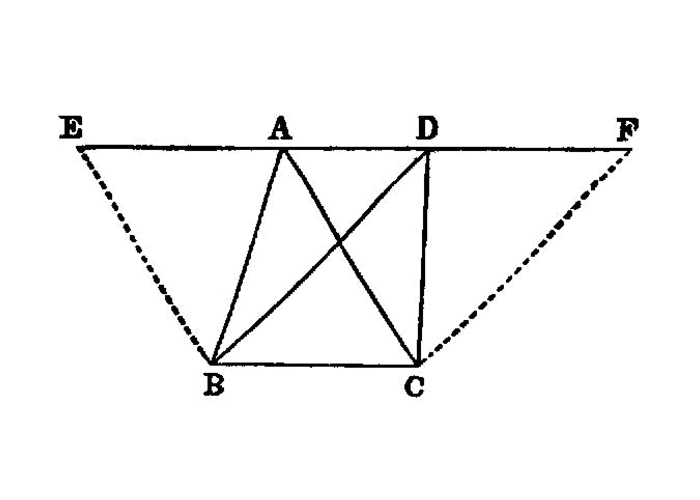
Triangles which are on the same base and in the same parallels are equal to one another.
Triangles which are on equal bases and in the same parallels are equal to one another.

Equal triangles which are on the same base and on the same side are also in the same parallels.

Equal triangles which are on equal bases and on the same side are also in the same parallels

If a parallelogram have the same base with a triangle and be in the same parallels, the parallelogram is double of the triangle.
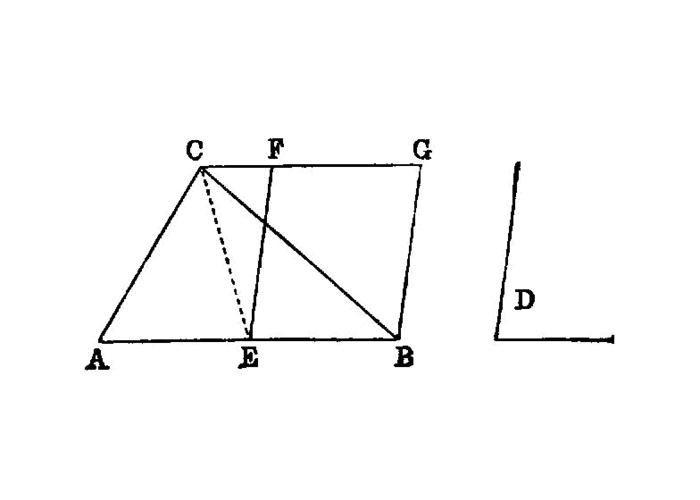
To construct, in a given rectilineal angle, a parallelogram equal to a given triangle.
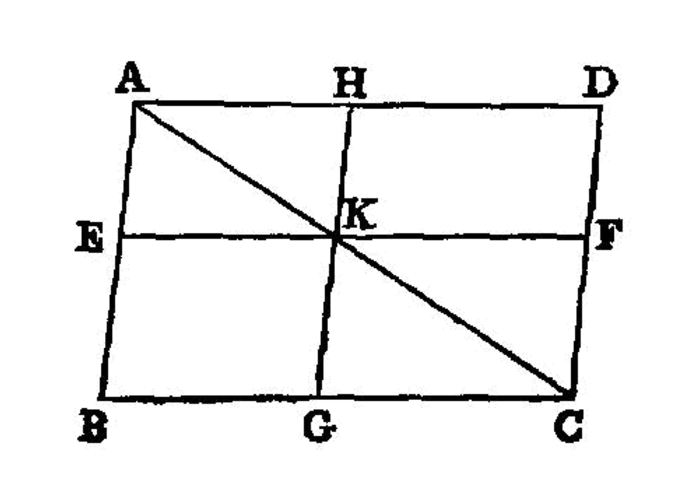
In any parallelogram the complements 1 of the parallelograms about the diameter are equal to one another.
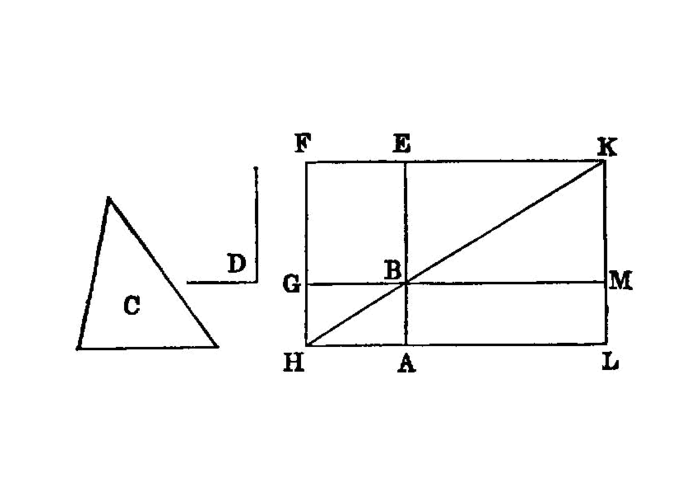
To a given straight line to apply, in a given rectilineal angle, a parallelogram equal to a given triangle.

To construct, in a given rectilineal angle, a parallelogram equal to a given rectilineal figure.
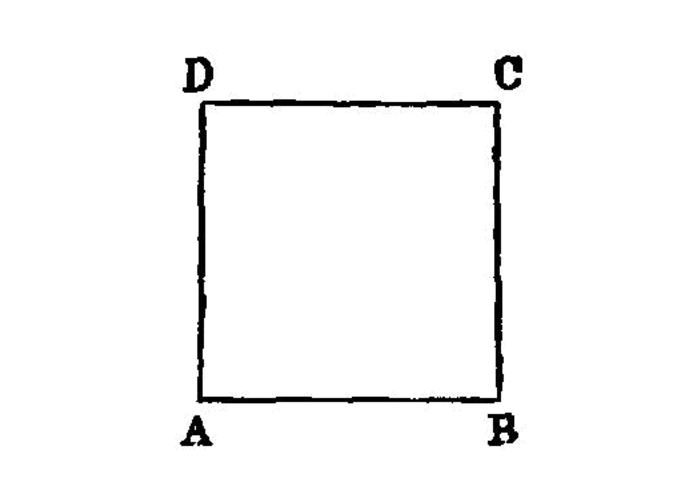
On a given straight line to describe a square.
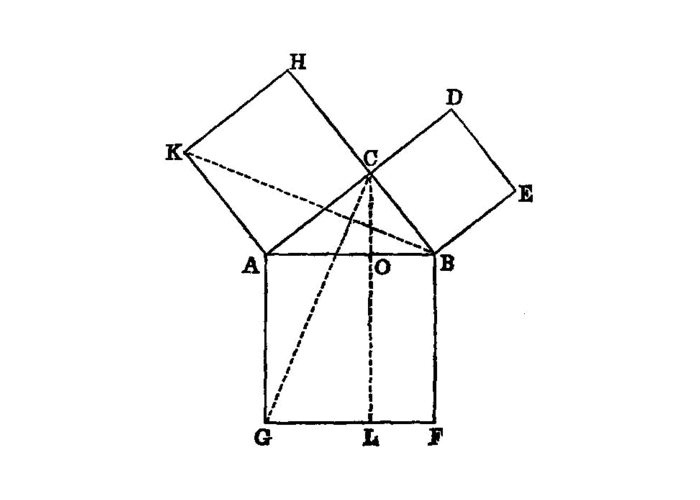

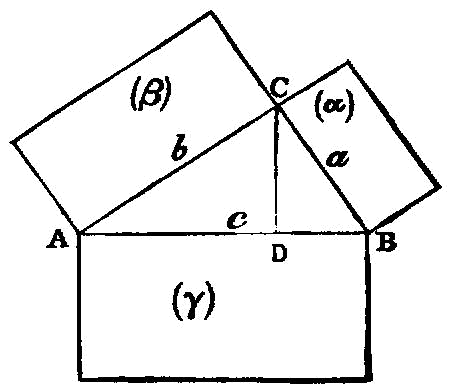
If in a triangle the square on one of the sides be equal to the squares on the remaining two sides of the triangle, the angle contained by the remaining two sides of the triangle is right.
Theory of Rectangles
Any rectangular parallelogram is said to be contained by the two straight lines containing the right angle.
And in any parallelogrammic area let any one whatever of the parallelograms about its diameter with the two complements be called a gnomon.
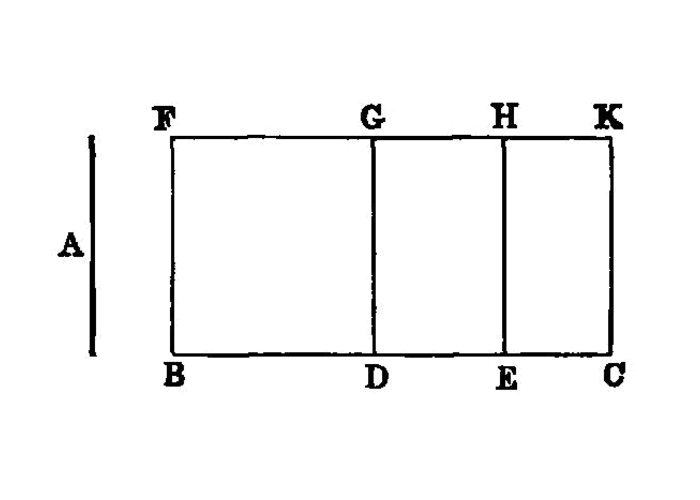
If there be two straight lines, and one of them be cut into any number of segments whatever, the rectangle contained by the two straight lines is equal to the rectangles contained by the uncut straight line and each of the segments.
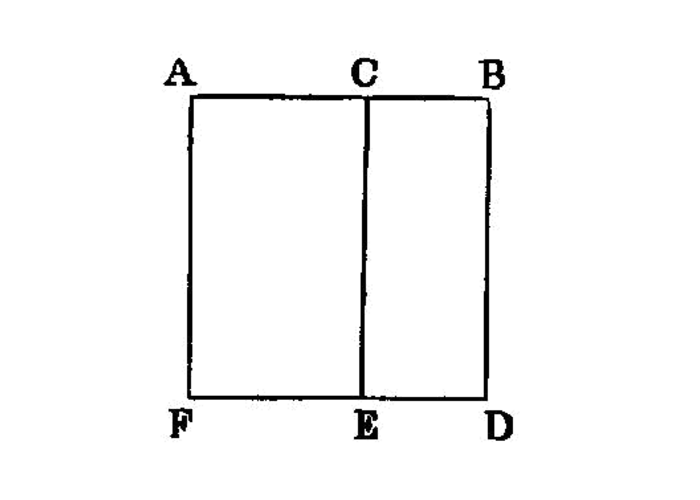
If a straight line be cut at random, the rectangle contained by the whole and both of the segments is equal to the square on the whole.

If a straight line be cut at random, the rectangle contained by the whole and one of the segments is equal to the rectangle contained by the segments and the square on the aforesaid segment.
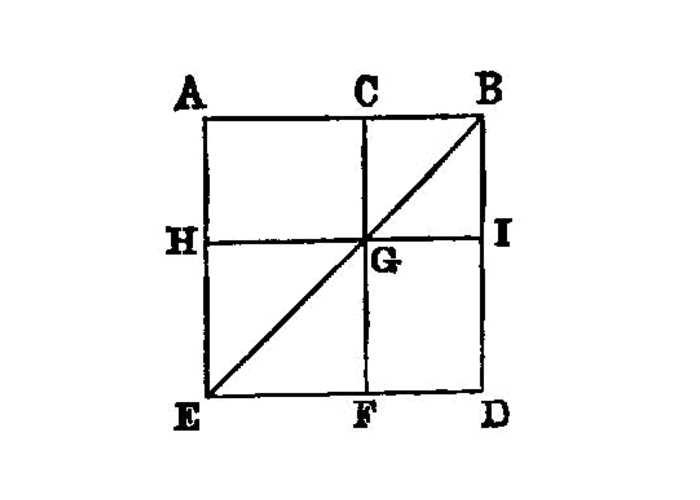
If a straight line be cut at random, the square on the whole is equal to the squares on the segments and twice the rectangle contained by the segments.
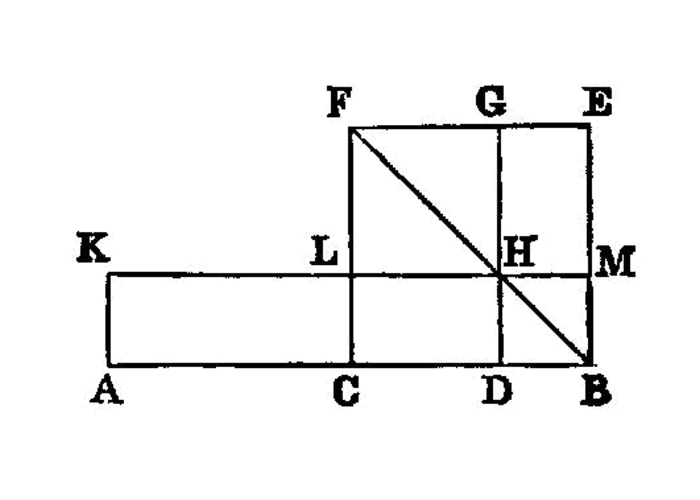
If a straight line be cut into equal and unequal segments, the rectangle contained by the unequal segments of the whole together with the square on the straight line between the points of section is equal to the square on the half.

If a straight line be bisected and a straight line be added to it in a straight line, the rectangle contained by the whole with the added straight line and the added straight line together with the square on the half is equal to the square on the straight line made up of the half and the added straight line.
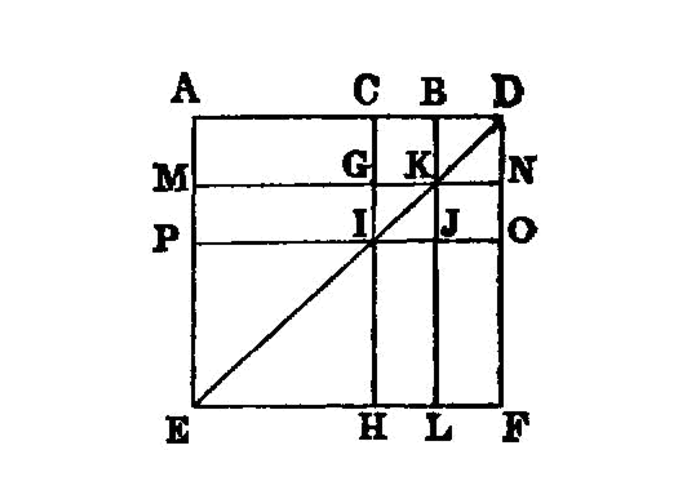
If a straight line be cut at random, the square on the whole and that on one of the segments both together are equal to twice the rectangle contained by the whole and the said segment and the square on the remaining segment.
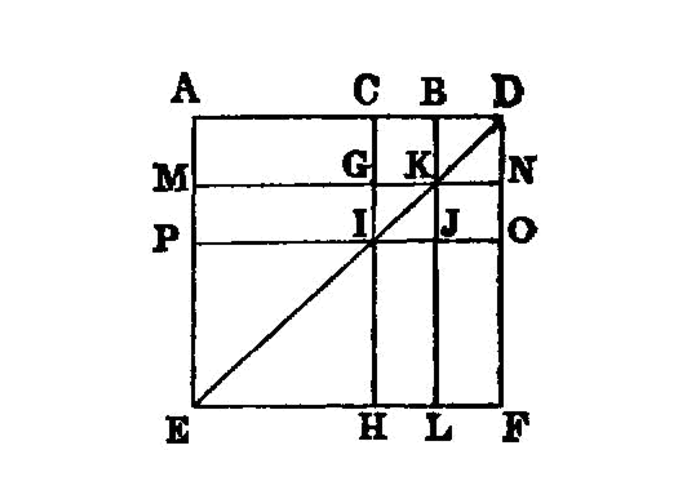
If a straight line be cut at random, four times the rectangle contained by the whole and one of the segments together with the square on the remaining segment is equal to the square described on the whole and the aforesaid segment as on one straight line.

If a straight line be cut into equal and unequal segments, the squares on the unequal segments of the whole are double of the square on the half and of the square on the straight line between the points of section.
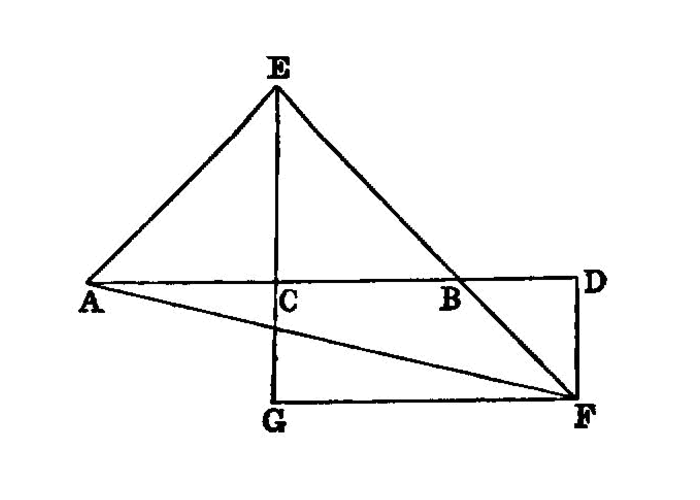
If a straight line be bisected, and a straight line be added to it in a straight line, the square on the whole with the added straight line and the square on the added straight line both together are double of the square on the half and of the square described on the straight line made up of the half and the added straight line as on one straight line.
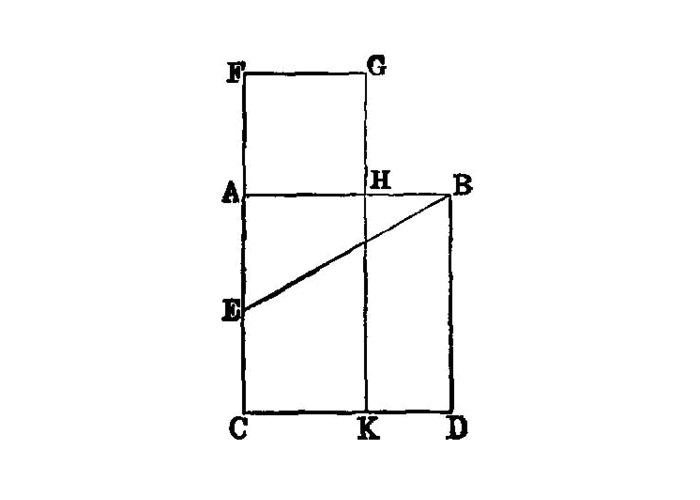
To cut a given straight line so that the rectangle contained by the whole and one of the segments is equal to the square on the remaining segment.

In obtuse-angled triangles the square on the side subtending the obtuse angle is greater than the squares on the sides containing the obtuse angle by twice the rectangle contained by one of the sides about the obtuse angle, namely that on which the

In acute-angled triangles the square on the side subtending the acute angle is less than the squares on the sides containing the acute angle by twice the rectangle contained by one of the sides about the acute angle, namely that on which the perpendicular falls, and the straight line cut off within by the perpendicular towards the acute angle.
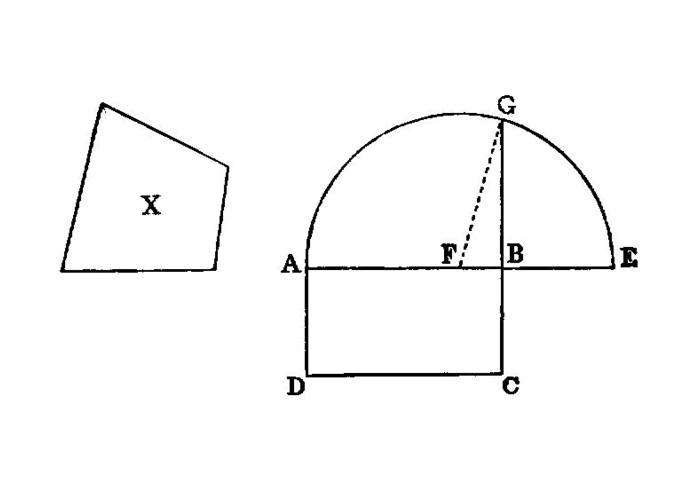
To construct a square equal to a given rectilineal figure.
Theory of the Circle
Equal circles are those the diameters of which are equal, or the radii of which are equal.
A straight line is said to touch a circle which, meeting the circle and being produced, does not cut the circle.
In a circle straight lines are said to be equally distant from the centre when the perpendiculars drawn to them from the centre are equal.
And that straight line is said to be at a greater distance on which the greater perpendicular falls.
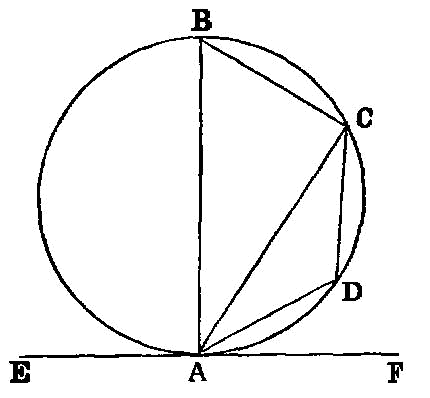
A segment of a circle is the figure contained by a straight line and a circumference of a circle.
An angle of a segment is that contained by a straight line and a circumference of a circle.
An angle in a segment is the angle which, when a point is taken on the circumference of the segment and straight lines are joined from it to the extremities of the straight line which is the base of the segment, is contained by the straight lines so joined.
And, when the straight lines containing the angle cut off a circumference, the angle is said to stand upon that circumference.
A sector of a circle is the figure which, when an angle is constructed at the centre of the circle, is contained by the straight lines containing the angle and the circumference cut off by them.
Similar segments of circles are those which admit equal angles, or in which the angles are equal to one another.




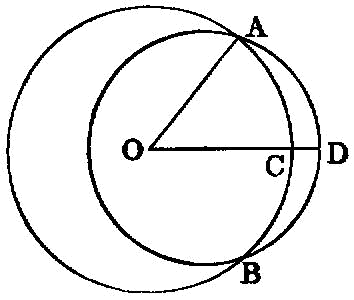




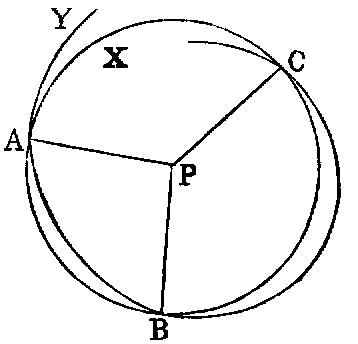
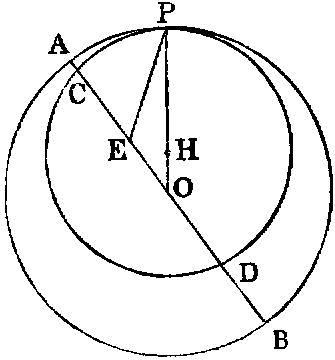


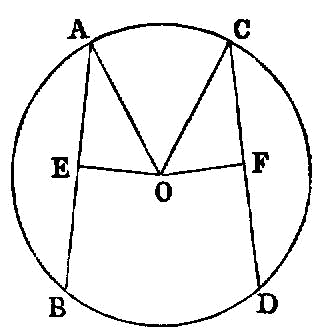
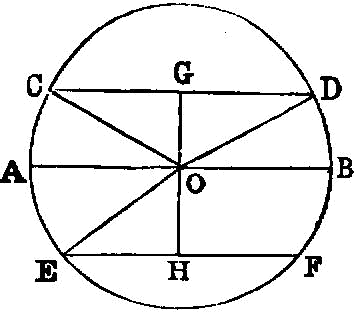
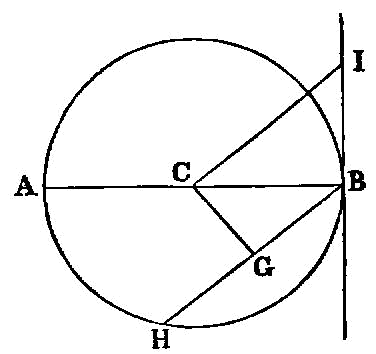
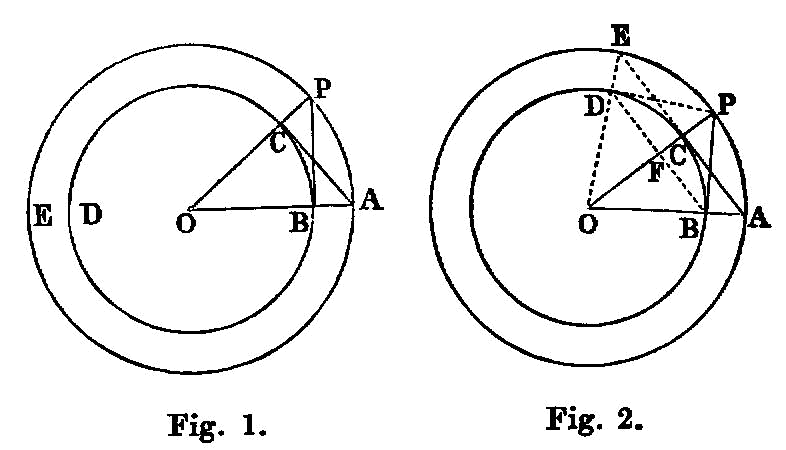
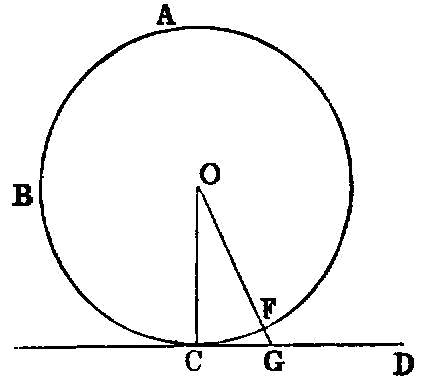


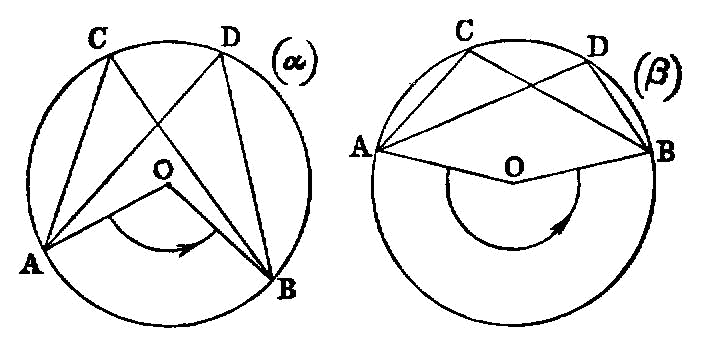
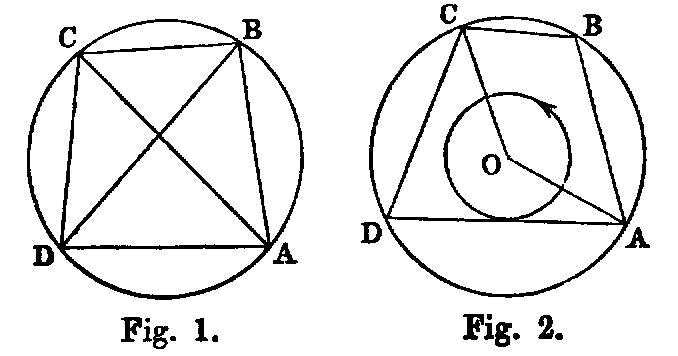

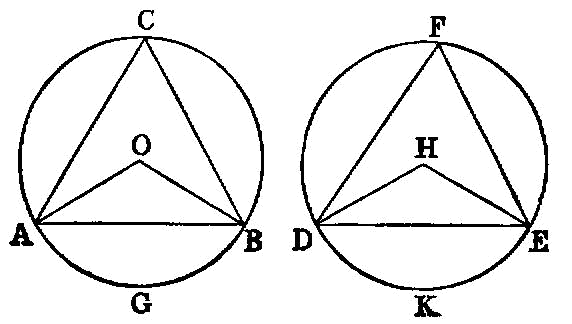
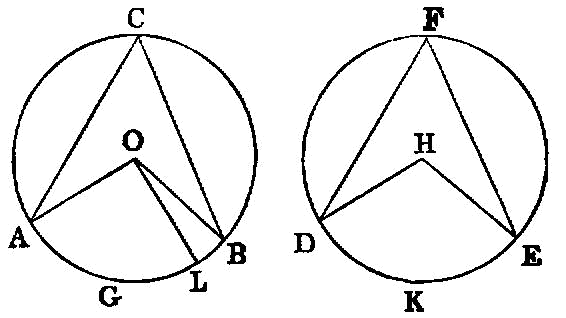
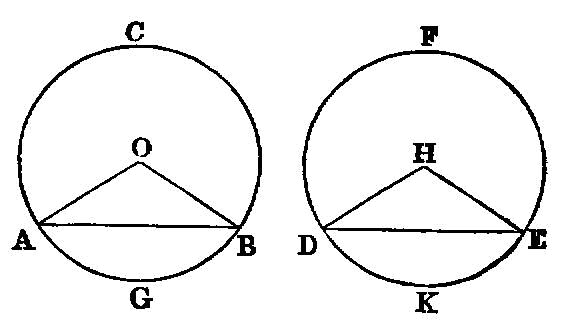
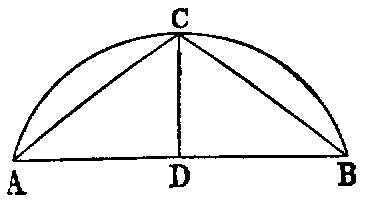
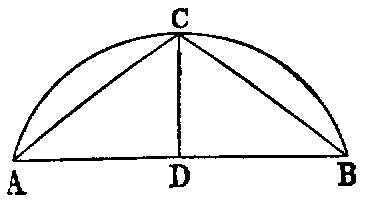

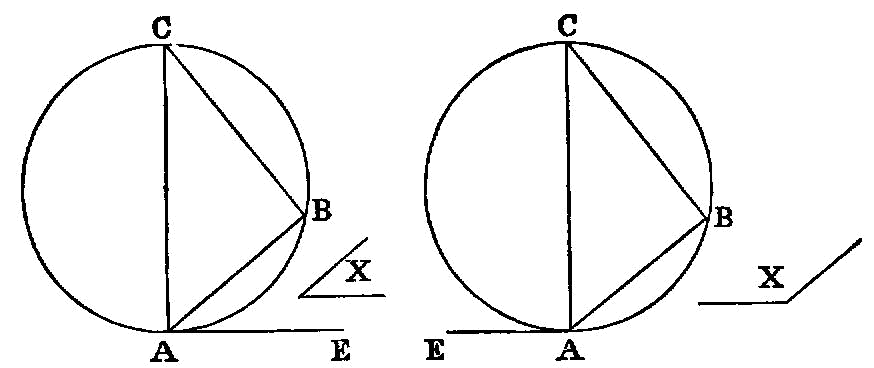

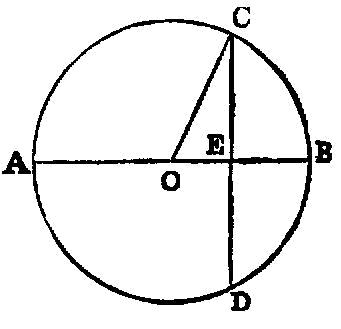
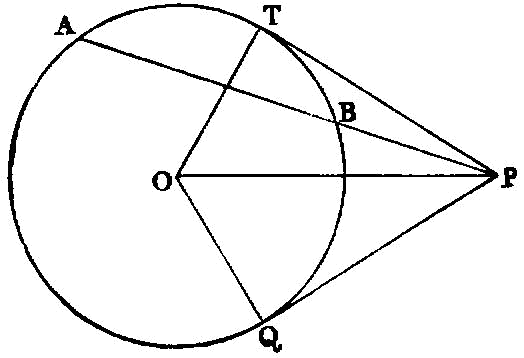
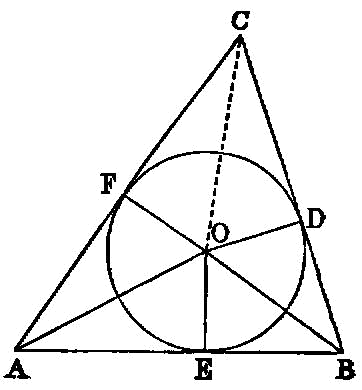
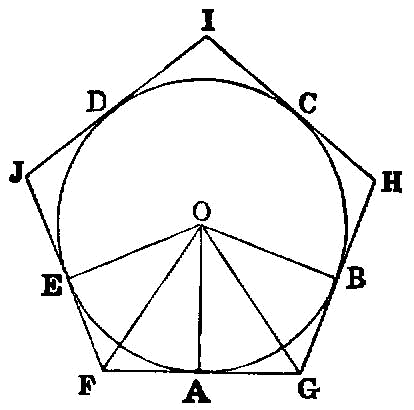
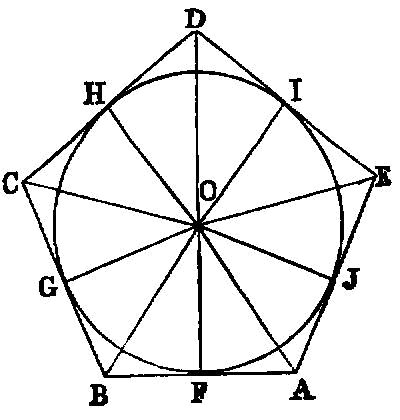
Inscription and circumscription of triangles and of regular polygons in and about circles
A rectilineal figure is said to be inscribed in a rectilineal figure when the respective angles of the inscribed figure lie on the respective sides of that in which it is inscribed.
Similarly a figure is said to be circumscribed about a figure when the respective sides of the circumscribed figure pass through the respective angles of that about which it is circumscribed.
A rectilineal figure is said to be inscribed in a circle when each angle of the inscribed figure lies on the circumference of the circle.
A rectilineal figure is said to be circumscribed about a circle, when each side of the circumscribed figure touches the circumference of the circle.
Similarly a circle is said to be inscribed in a figure when the circumference of the circle touches each side of the figure in which it is inscribed.
A circle is said to be circumscribed about a figure when the circumference of the circle passes through each angle of the figure about which it is circumscribed.
A straight line is said to be fitted into a circle when its extremities are on the circumference of the circle.
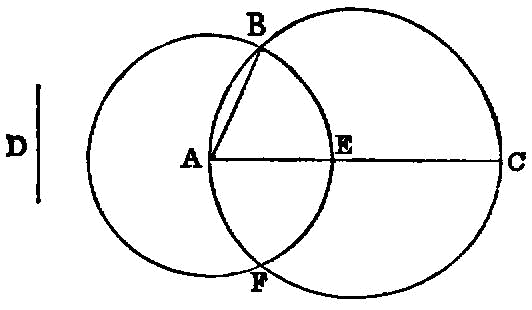
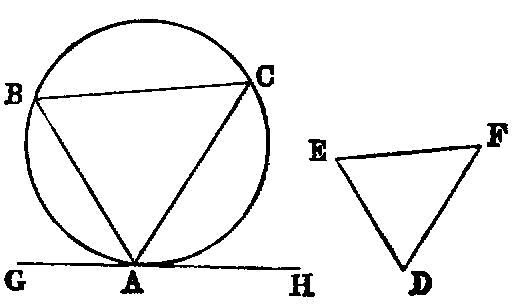
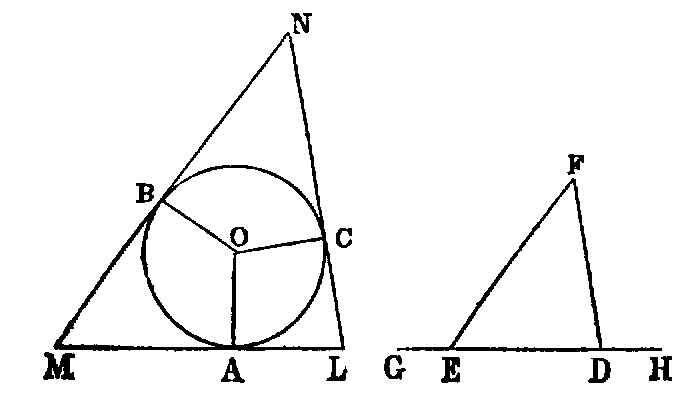








Theory of Proportion
A magnitude is a part of a magnitude, the less of the greater, when it measures the greater.
The greater is a multiple of the less when it is measured by the less.
A ratio is a sort of relation in respect of size between two magnitudes of the same kind.
Magnitudes are said to have a ratio to one another which are capable, when multiplied, of exceeding one another.
Magnitudes are said to be in the same ratio, the first to the second and the third to the fourth, when, if any equimultiples whatever be taken of the first and third, and any equimultiples whatever of the second and fourth, the former equimultiples alike exceed, are alike equal to, or alike fall short of, the latter equimultiples respectively taken in corresponding order.
Let magnitudes which have the same ratio be called proportional.
When, of the equimultiples, the multiple of the first magnitude exceeds the multiple of the second, but the multiple of the third does not exceed the multiple of the fourth, then the first is said to have a greater ratio to the second than the third has to the fourth.
A proportion in three terms is the least possible.
When three magnitudes are proportional, the first is said to have to the third the duplicate ratio of that which it has to the second.
When four magnitudes are <continuously> proportional, the first is said to have to the fourth the triplicate ratio of that which it has to the second, and so on continually, whatever be the proportion.
The term corresponding magnitudes is used of antecedents in relation to antecedents, and of consequents in relation to consequents.
Alternate ratio means taking the antecedent in relation to the antecedent and the consequent in relation to the consequent.
Inverse ratio means taking the consequent as antecedent in relation to the antecedent as consequent.
Composition of a ratio means taking the antecedent together with the consequent as one in relation to the consequent by itself.
Separation of a ratio means taking the excess by which the antecedent exceeds the consequent in relation to the consequent by itself.
Conversion of a ratio means taking the antecedent in relation to the excess by which the antecedent exceeds the consequent.
A ratio
A perturbed proportion arises when, there being three magnitudes and another set equal to them in multitude, as antecedent is to consequent among the first magnitudes, so is antecedent to consequent among the second magnitudes, while, as the consequent is to a third among the first magnitudes, so is a third to the antecedent among the second magnitudes.
Application of the theory of proportion
Similar rectilineal figures are such as have their angles severally equal and the sides about the equal angles proportional.
[Reciprocally related figures.
A straight line is said to have been cut in extreme and mean ratio when, as the whole line is to the greater segment, so is the greater to the less.
The height of any figure is the perpendicular drawn from the vertex to the base.

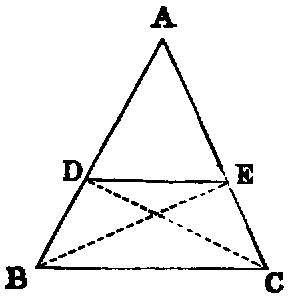



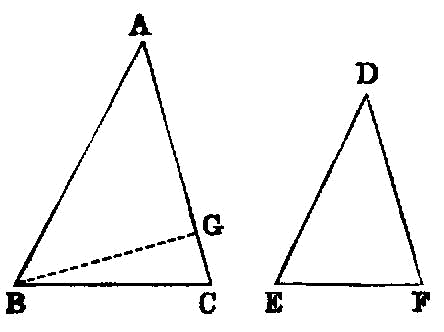






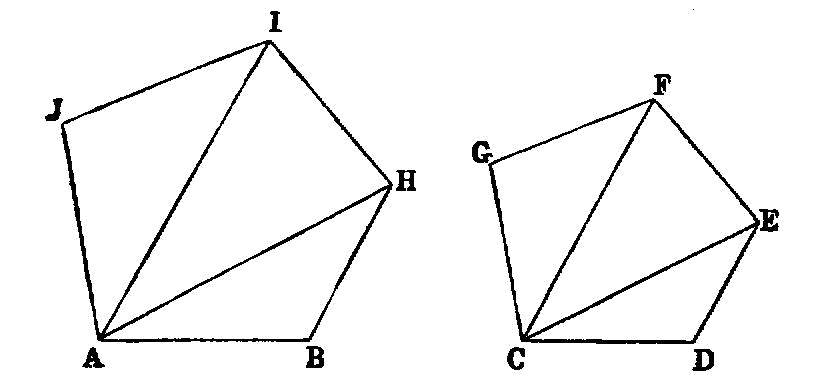
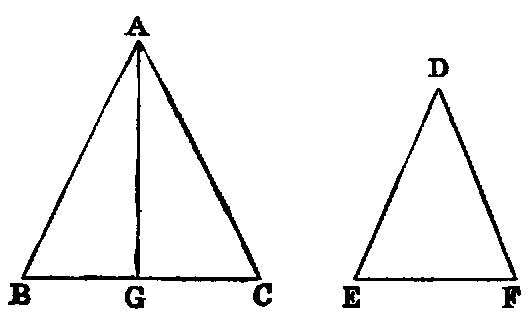
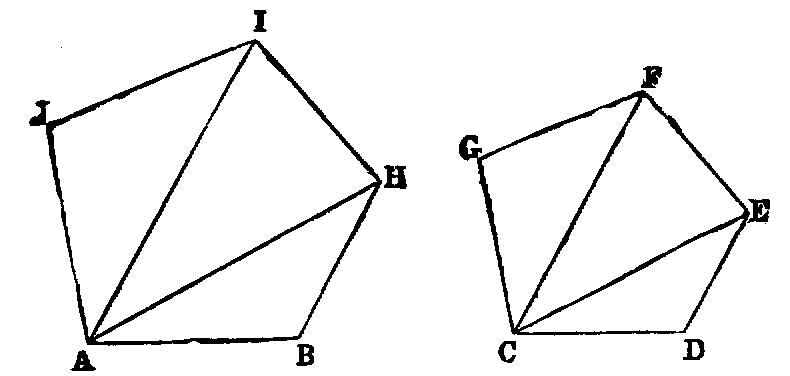
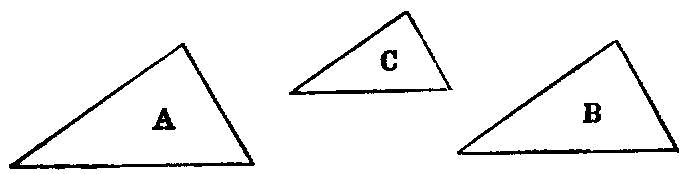
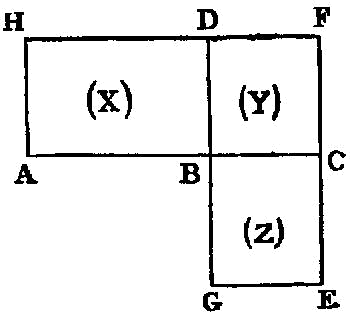
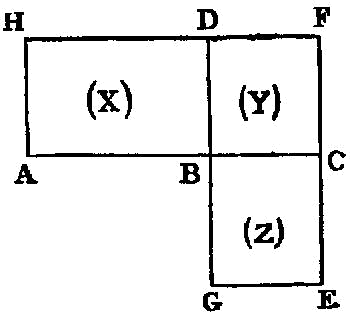
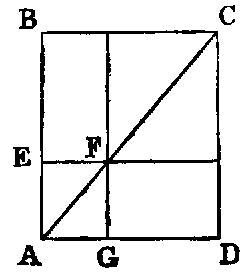
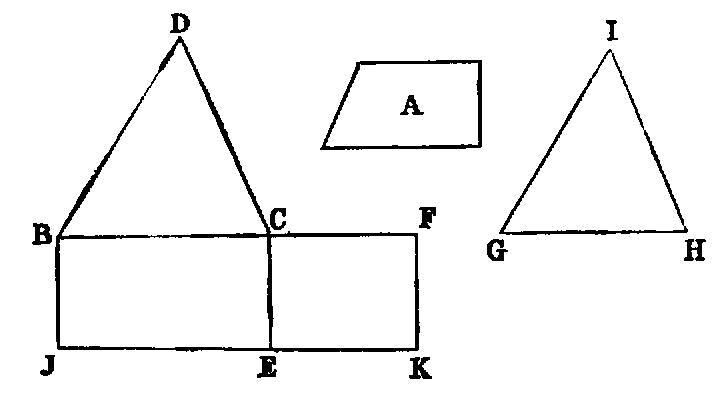
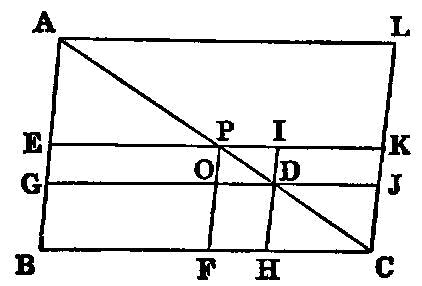


To cut a given finite straight line in extreme and mean ratio.
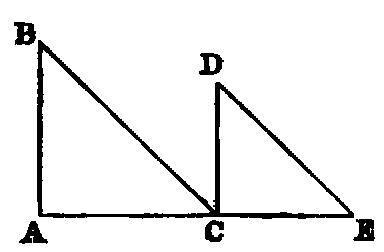
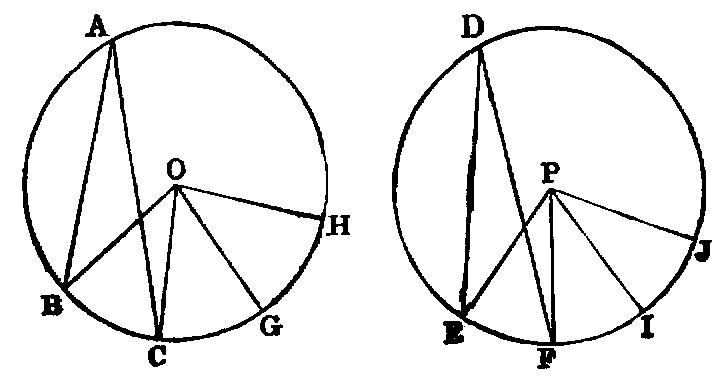
An unit is that by virtue of which each of the things that exist is called one.
A number is a multitude composed of units.
A number is a part of a number, the less of the greater, when it measures the greater;
but parts when it does not measure it.
The greater number is a multiple of the less when it is measured by the less.
An even number is that which is divisible into two equal parts.
An odd number is that which is not divisible into two equal parts, or that which differs by an unit from an even number.
An even-times even number is that which is measured by an even number according to an even number.
An even-times odd number is that which is measured by an even number according to an odd number.
An odd-times odd number is that which is measured by an odd number according to an odd number.
A prime number is that which is measured by an unit alone.
Numbers prime to one another are those which are measured by an unit alone as a common measure.
A composite number is that which is measured by some number.
Numbers composite to one another are those which are measured by some number as a common measure.
A number is said to multiply a number when that which is multiplied is added to itself as many times as there are units in the other, and thus some number is produced.
And, when two numbers having multiplied one another make some number, the number so produced is called plane, and its sides are the numbers which have multiplied one another.
And, when three numbers having multiplied one another make some number, the number so produced is solid, and its sides are the numbers which have multiplied one another.
A square number is equal multiplied by equal, or a number which is contained by two equal numbers.
And a cube is equal multiplied by equal and again by equal, or a number which is contained by three equal numbers.
Numbers are proportional when the first is the same multiple, or the same part, or the same parts, of the second that the third is of the fourth.
Similar plane and solid numbers are those which have their sides proportional.
A perfect number is that which is equal to its own parts.
Two unequal numbers being set out, and the less being continually subtracted in turn from the greater, if the number which is left never measures the one before it until an unit is left, the original numbers will be prime to one another.
Given two numbers not prime to one another, to find their greatest common measure.
Given three numbers not prime to one another, to find their greatest common measure.
Any number is either a part or parts of any number, the less of the greater.
If a number be a part of a number, and another be the same part of another, the sum will also be the same part of the sum that the one is of the one.
If a number be parts of a number, and another be the same parts of another, the sum will also be the same parts of the sum that the one is of the one.
If a number be that part of a number, which a number subtracted is of a number subtracted, the remainder will also be the same part of the remainder that the whole is of the whole.
If a number be the same parts of a number that a number subtracted is of a number subtracted, the remainder will also be the same parts of the remainder that the whole is of the whole.
If a number be a part of a number, and another be the same part of another, alternately also, whatever part or parts the first is of the third, the same part, or the same parts, will the second also be of the fourth.
If a number be parts of a number, and another be the same parts of another, alternately also, whatever parts or part the first is of the third, the same parts or the same part will the second also be of the fourth.
If, as whole is to whole, so is a number subtracted to a number subtracted, the remainder will also be to the remainder as whole to whole.
If there be as many numbers as we please in proportion, then, as one of the antecedents is to one of the consequents, so are all the antecedents to all the consequents.
If four numbers be proportional, they will also be proportional alternately.
If there be as many numbers as we please, and others equal to them in multitude, which taken two and two are in the same ratio, they will also be in the same ratio ex aequali.
If an unit measure any number, and another number measure any other number the same number of times, alternately also, the unit will measure the third number the same number of times that the second measures the fourth.
If two numbers by multiplying one another make certain numbers, the numbers so produced will be equal to one another.
If a number by multiplying two numbers make certain numbers, the numbers so produced will have the same ratio as the numbers multiplied.
If two numbers by multiplying any number make certain numbers, the numbers so produced will have the same ratio as the multipliers.
If four numbers be proportional, the number produced from the first and fourth will be equal to the number produced from the second and third; and, if the number produced from the first and fourth be equal to that produced from the second and third, the four numbers will be proportional.
The least numbers of those which have the same ratio with them measure those which have the same ratio the same number of times, the greater the greater and the less the less.
Numbers prime to one another are the least of those which have the same ratio with them.
The least numbers of those which have the same ratio with them are prime to one another.
If two number be prime to one another, the number which measures the one of them will be prime to the remaining number.
If two numbers be prime to any number, their product also will be prime to the same.
If two numbers be prime to one another, the product of one of them into itself will be prime to the remaining one.
If two numbers be prime to two numbers, both to each, their products also will be prime to one another.
If two numbers be prime to one another, and each by multiplying itself make a certain number, the products will be prime to one another; and, if the original numbers by multiplying the products make certain numbers, the latter will also be prime to one another [and this is always the case with the extremes].
If two numbers be prime to one another, the sum will also be prime to each of them; and, if the sum of two numbers be prime to any one of them, the original numbers will also be prime to one another.
Any prime number is prime to any number which it does not measure.
If two numbers by multiplying one another make some number, and any prime number measure the product, it will also measure one of the original numbers.
Any composite number is measured by some prime number.
Any number either is prime or is measured by some prime number.
Given as many numbers as we please, to find the least of those which have the same ratio with them.
Given two numbers, to find the least number which they measure.
If two numbers measure any number, the least number measured by them will also measure the same.
Given three numbers, to find the least number which they measure.
If a number be measured by any number, the number which is measured will have a part called by the same name as the measuring number.
If a number have any part whatever, it will be measured by a number called by the same name as the part.
To find the number which is the least that will have given parts.
If there be as many numbers as we please in continued proportion, and the extremes of them be prime to one another, the numbers are the least of those which have the same ratio with them.
To find numbers in continued proportion, as many as may be prescribed, and the least that are in a given ratio.
If as many numbers as we please in continued proportion be the least of those which have the same ratio with them, the extremes of them are prime to one another.
Given as many ratios as we please in least numbers, to find numbers in continued proportion which are the least in the given ratios.
Plane numbers have to one another the ratio compounded of the ratios of their sides.
If there be as many numbers as we please in continued proportion, and the first do not measure the second, neither will any other measure any other.
If there be as many numbers as we please in continued proportion, and the first measure the last, it will measure the second also.
If between two numbers there fall numbers in continued proportion with them, then, however many numbers fall between them in continued proportion, so many will also fall in continued proportion between the numbers which have the same ratio with the original numbers.
If two numbers be prime to one another, and numbers fall between them in continued proportion, then, however many numbers fall between them in continued proportion, so many will also fall between each of them and an unit in continued proportion.
If numbers fall between each of two numbers and an unit in continued proportion, however many numbers fall between each of them and an unit in continued proportion, so many also will fall between the numbers themselves in continued proportion.
Between two square numbers there is one mean proportional number, and the square has to the square the ratio duplicate of that which the side has to the side.
Between two cube numbers there are two mean proportional numbers, and the cube has to the cube the ratio triplicate of that which the side has to the side.
If there be as many numbers as we please in continued proportion, and each by multiplying itself make some number, the products will be proportional; and, if the original numbers by multiplying the products make certain numbers, the latter will also be proportional.
If a square measure a square, the side will also measure the side; and, if the side measure the side, the square will also measure the square.
If a cube number measure a cube number, the side will also measure the side; and, if the side measure the side, the cube will also measure the cube.
If a square number do not measure a square number, neither will the side measure the side; and, if the side do not measure the side, neither will the square measure the square.
If a cube number do not measure a cube number, neither will the side measure the side; and, if the side do not measure the side, neither will the cube measure the cube.
Between two similar plane numbers there is one mean proportional number; and the plane number has to the plane number the ratio duplicate of that which the corresponding side has to the corresponding side.
Between two similar solid numbers there fall two mean proportional numbers; and the solid number has to the similar solid number the ratio triplicate of that which the corresponding side has to the corresponding side.
If one mean proportional number fall between two numbers, the numbers will be similar plane numbers.
If two mean proportional numbers fall between two numbers, the numbers are similar solid numbers.
If three numbers be in continued proportion, and the first be square, the third will also be square.
If four numbers be in continued proportion, and the first be cube, the fourth will also be cube.
If two numbers have to one another the ratio which a square number has to a square number, and the first be square, the second will also be square.
If two numbers have to one another the ratio which a cube number has to a cube number, and the first be cube, the second will also be cube.
Similar plane numbers have to one another the ratio which a square number has to a square number.
Similar solid numbers have to one another the ratio which a cube number has to a cube number.
If two similar plane numbers by multiplying one another make some number, the product will be square.
If two numbers by multiplying one another make a square number, they are similar plane numbers.
If a cube number by multiplying itself make some number, the product will be cube.
If a cube number by multiplying a cube number make some number, the product will be cube.
If a cube number by multiplying any number make a cube number, the multiplied number will also be cube.
If a number by multiplying itself make a cube number, it will itself also be cube.
If a composite number by multiplying any number make some number, the product will be solid.
If as many numbers as we please beginning from an unit be in continued proportion, the third from the unit will be square, as will also those which successively leave out one; the fourth will be cube, as will also all those which leave out two; and the seventh will be at once cube and square, as will also those which leave out five.
If as many numbers as we please beginning from an unit be in continued proportion, and the number after the unit be square, all the rest will also be square. And, if the number after the unit be cube, all the rest will also be cube.
If as many numbers as we please beginning from an unit be in continued proportion, and the number after the unit be not square, neither will any other be square except the third from the unit and all those which leave out one. And, if the number after the unit be not cube, neither will any other be cube except the fourth from the unit and all those which leave out two.
If as many numbers as we please beginning from an unit be in continued proportion, the less measures the greater according to some one of the numbers which have place among the proportional numbers.
If as many numbers as we please beginning from an unit be in continued proportion, by however many prime numbers the last is measured, the next to the unit will also be measured by the same.
If as many numbers as we please beginning from an unit be in continued proportion, and the number after the unit be prime, the greatest will not be measured by any except those which have a place among the proportional numbers.
If a number be the least that is measured by prime numbers, it will not be measured by any other prime number except those originally measuring it.
If three numbers in continued proportion be the least of those which have the same ratio with them, any two whatever added together will be prime to the remaining number.
If two numbers be prime to one another, the second will not be to any other number as the first is to the second.
If there be as many numbers as we please in continued proportion, and the extremes of them be prime to one another, the last will not be to any other number as the first to the second.
Given two numbers, to investigate whether it is possible to find a third proportional to them.
Given three numbers, to investigate when it is possible to find a fourth proportional to them.
Prime numbers are more than any assigned multitude of prime numbers.
If as many even numbers as we please be added together, the whole is even.
If as many odd numbers as we please be added together, and their multitude be even, the whole will be even.
If as many odd numbers as we please be added together, and their multitude be odd, the whole will also be odd.
If from an even number an even number be subtracted, the remainder will be even.
If from an even number an odd number be subtracted, the remainder will be odd.
If from an odd number an odd number be subtracted, the remainder will be even.
If from an odd number an even number be subtracted, the remainder will be odd.
If an odd number by multiplying an even number make some number, the product will be even.
If an odd number by multiplying an odd number make some number, the product will be odd.
If an odd number measure an even number, it will also measure the half of it.
If an odd number be prime to any number, it will also be prime to the double of it.
Each of the numbers which are continually doubled beginning from a dyad is even-times even only.
If a number have its half odd, it is even-times odd only.
If a number neither be one of those which are continually doubled from a dyad, nor have its half odd, it is both eventimes even and even-times odd.
If as many numbers as we please be in continued proportion, and there be subtracted from the second and the last numbers equal to the first, then, as the excess of the second is to the first, so will the excess of the last be to all those before it.
If as many numbers as we please beginning from an unit be set out continuously in double proportion, until the sum of all becomes prime, and if the sum multiplied into the last make some number, the product will be perfect.
Those magnitudes are said to be commensurable which are measured by the same measure, and those incommensurable which cannot have any common measure.
Straight lines are commensurable in square when the squares on them are measured by the same area, and incommensurable in square when the squares on them cannot possibly have any area as a common measure.
With these hypotheses, it is proved that there exist straight lines infinite in multitude which are commensurable and incommensurable respectively, some in length only, and others in square also, with an assigned straight line. Let then the assigned straight line be called rational, and those straight lines which are commensurable with it, whether in length and in square or in square only, rational, but those which are incommensurable with it irrational.
And let the square on the assigned straight line be called rational and those areas which are commensurable with it rational, but those which are incommensurable with it irrational, and the straight lines which produce them irrational, that is, in case the areas are squares, the sides themselves, but in case they are any other rectilineal figures, the straight lines on which are described squares equal to them.
Two unequal magnitudes being set out, if from the greater there be subtracted a magnitude greater than its half, and from that which is left a magnitude greater than its half, and if this process be repeated continually, there will be left some magnitude which will be less than the lesser magnitude set out.
If, when the less of two unequal magnitudes is continually subtracted in turn from the greater, that which is left never measures the one before it, the magnitudes will be incommensurable.
Given two commensurable magnitudes, to find their greatest common measure.
Given three commensurable magnitudes, to find their greatest common measure.
Commensurable magnitudes have to one another the ratio which a number has to a number.
If two magnitudes have to one another the ratio which a number has to a number, the magnitudes will be commensurable.
Incommensurable magnitudes have not to one another the ratio which a number has to a number.
If two magnitudes have not to one another the ratio which a number has to a number, the magnitudes will be incommensurable.
The squares on straight lines commensurable in length have to one another the ratio which a square number has to a square number; and squares which have to one another the ratio which a square number has to a square number will also have their sides commensurable in length.
To find two straight lines incommensurable, the one in length only, and the other in square also, with an assigned straight line.
If four magnitudes be proportional, and the first be commensurable with the second, the third will also be commensurable with the fourth; and, if the first be incommensurable with the second, the third will also be incommensurable with the fourth.
Magnitudes commensurable with the same magnitude are commensurable with one another also.
If two magnitudes be commensurable, and the one of them be incommensurable with any magnitude, the remaining one will also be incommensurable with the same.
If four straight lines be proportional, and the square on the first be greater than the square on the second by the square on a straight line commensurable with the first, the square on the third will also be greater than the square on the fourth by
the square on a straight line commensurable with the third.
If two commensurable magnitudes be added together, the whole will also be commensurable with each of them; and, if the whole be commensurable with one of them, the original magnitudes will also be commensurable.
If two incommensurable magnitudes be added together, the whole will also be incommensurable with each of them; and, if the whole be incommensurable with one of them, the original magnitudes will also be incommensurable.
If there be two unequal straight lines, and to the greater there be applied a parallelogram equal to the fourth part of the square on the less and deficient by a square figure, and if it divide it into parts which are commensurable in length, then the square on the greater will be greater than the square on the less by the square on a straight line commensurable with the greater.
If there be two unequal straight lines, and to the greater there be applied a parallelogram equal to the fourth part of the square on the less and deficient by a square figure, and if it divide it into parts which are incommensurable, the square on the greater will be greater than the square on the less by the square on a straight line incommensurable with the greater.
The rectangle contained by rational straight lines commensurable in length is rational.
If a rational area be applied to a rational straight line, it produces as breadth a straight line rational and commensurable in length with the straight line to which it is applied.
The rectangle contained by rational straight lines commensurable in square only is irrational, and the side of the square equal to it is irrational. Let the latter be called
medial.
The square on a medial straight line, if applied to a rational straight line, produces as breadth a straight line rational and incommensurable in length with that to which it is applied.
A straight line commensurable with a medial straight line is medial.
The rectangle contained by medial straight lines commensurable in length is medial.
The rectangle contained by medial straight lines commensurable in square only is either rational or medial.
4 medial area does not exceed a medial area by a rational area.
To find medial straight lines commensurable in square only which contain a rational rectangle.
To find medial straight lines commensurable in square only which contain a medial rectangle.
To find two rational straight lines commensurable in square only and such that the square on the greater is greater than the square on the less by the square on a straight line commensurable in length with the greater.
To find two rational straight lines commensurable in square only and such that the square on the greater is greater is greater than the square on the less by the square on a straight line incommensurable in length with the greater.
To find two medial straight lines commensurable in square only, containing a rational rectangle, and such that the square on the greater is greater than the square on the less by the square on a straight line commensurable in length with the greater.
To find two medial straight lines commensurable in square only, containing a medial rectangle, and such that the square on the greater is greater than the square on the less by the square on a straight line commensurable with the greater.
To find two straight lines incommensurable in square which make the sum of the squares on them rational but the rectangle contained by them medial.
To find two straight lines incommensurable in square which make the sum of the squares on them medial but the rectangle contained by them rational.
To find two straight lines incommensurable in square which make the sum of the squares on them medial and the rectangle contained by them medial and moreover incommensurable with the sum of the squares on them.
If two rational straight lines commensurable in square only be added together, the whole is irrational; and let it be called
binomial.
If two medial straight lines commensurable in square only and containing a rational rectangle be added together, the whole is irrational; and let it be called
a first bimedial
straight line.
If two medial straight lines commensurable in square only and containing a medial rectangle be added together, the whole is irrational; and let it be called a
second bimedial
straight line.
If two straight lines incommensurable in square which make the sum of the squares on them rational, but the rectangle contained by them medial, be added together, the whole straight line is irrational : and let it be called
major.
If two straight lines incommensurable in square which make the sum of the squares on them medial, but the rectangle contained by them rational, be added together, the whole straight line is irrational; and let it be called the
side of a rational plus a medial area.
If two straight lines incommensurable in square which make the sum of the squares on them medial, and the rectangle contained by them medial and also incommensurable with the sum of the squares on them, be added together, the whole straight line is irrational; and let it be called the
side of the sum of two medial areas.
A binomial straight line is divided into its terms at one point only.
A first bimedial straight line is divided at one point only.
A second bimedial straight line is divided at one point only.
A major straight line is divided at one and the same point only.
The side of a rational plus a medial area is divided at one point only.
The side of the sum of two medial areas is divided at one point only.
Given a rational straight line and a binomial, divided into its terms, such that the square on the greater term is greater than the square on the lesser by the square on a straight line commensurable in length with the greater, then, if the greater term be commensurable in length with the rational straight line set out, let the whole be called a first binomial straight line;
but if the lesser term be commensurable in length with the rational straight line set out, let the whole be called a second binomial;
and if neither of the terms be commensurable in length with the rational straight line set out, let the whole be called a third binomial.
Again, if the square on the greater term be greater than the square on the lesser by the square on a straight line incommensurable in length with the greater, then, if the greater term be commensurable in length with the rational straight line set out, let the whole be called a fourth binomial;
if the lesser, a fifth binomial;
and if neither, a sixth binomial.
To find the first binomial straight line.
To find the second binomial straight line.
To find the third binomial straight line.
To find the fourth binomial straight line.
To find the fifth binomial straight line.
To find the sixth binomial straight line.
If an area be contained by a rational straight line and the first binomial, the side
of the area is the irrational straight line which is called binomial.
If an area be contained by a rational straight line and the second binomial, the side
of the area is the irrational straight line which is called a first bimedial.
If an area be contained by a rational straight line and the third binomial, the side
of the area is the irrational straight line called a second bimedial.
If an area be contained by a rational straight line and the fourth binomial, the side
of the area is the irrational straight line called major.
If an area be contained by a rational straight line and the fifth binomial, the side
of the area is the irrational straight line called the side of a rational plus a medial area.
If an area be contained by a rational straight line and the sixth binomial, the side
of the area is the irrational straight line called the side of the sum of two medial areas.
The square on the binomial straight line applied to a rational straight line produces as breadth the first binomial.
The square on the first bimedial straight line applied to a rational straight line produces as breadth the second binomial.
The square on the second bimedial straight line applied to a rational straight line produces as breadth the third binomial.
The square on the major straight line applied to a rational straight line produces as breadth the fourth binomial.
The square on the side of a rational plus a medial area applied to a rational straight line produces as breadth the fifth binomial.
The square on the side of the sum of two medial areas applied to a rational straight line produces as breadth the sixth binomial.
A straight line commensurable in length with a binomial straight line is itself also binomial and the same in order.
A straight line commensurable in length with a bimedial straight line is itself also bimedial and the same in order.
A straight line commensurable with a major straight line is itself also major.
A straight line commensurable with the side of a rational plus a medial area is itself also the side of a rational plus a medial area.
A straight line commensurable with the side of the sum of two medial areas is the side of the sum of two medial areas.
If a rational and a medial area be added together, four irrational straight lines arise, namely a binomial or a first bimedial or a major or a side of a rational plus a medial area.
If two medial areas incommensurable with one another be added together, the remaining two irrational straight lines arise, namely either a second bimedial or a side of the sum of two medial areas.
If from a rational straight line there be subtracted a rational straight line commensurable with the whole in square only, the remainder is irrational; and let it be called
an apotome.
If from a medial straight line there be subtracted a medial straight line which is commensurable with the whole in square only, and which contains with the whole a rational rectangle, the remainder is irrational. And let it be called a
first apotome of a medial
straight line.
If from a medial straight line there be subtracted a medial straight line which is commensurable with the whole in square only, and which contains with the whole a medial rectangle, the remainder is irrational; and let it be called a
second apotome of a medial
straight line.
If from a straight line there be subtracted a straight line which is incommensurable in square with the whole and which with the whole makes the squares on them added together rational, but the rectangle contained by them medial, the remainder is irrational; and let it be called
minor.
If from a straight line there be subtracted a straight line which is incommensurable in square with the whole, and which with the whole makes the sum of the squares on them medial, but twice the rectangle contained by them rational, the remainder is irrational: and let it be called
that which produces with a rational area a medial whole.
If from a straight line there be subtracted a straight line which is incommensurable in square with the whole and which with the whole makes the sum of the squares on them medial, twice the rectangle contained by them medial, and further the squares on them incommensurable with twice the rectangle contained by them, the remainder is irrational; and let it be called
that which produces with a medial area a medial whole.
To an apotome only one rational straight line can be annexed which is commensurable with the whole in square only.
To a first apotome of a medial straight line only one medial straight line can be annexed which is commensurable with the whole in square only and which contains with the whole a rational rectangle.
To a second apotome of a medial straight line only one medial straight line can be annexed which is commensurable with the whole in square only and which contains with the whole a medial rectangle.
To a minor straight line only one straight line can be annexed which is incommensurable in square with the whole and which makes, with the whole, the sum of the squares on them rational but twice the rectangle contained by them medial.
To a straight line which produces with a rational area a medial whole only one straight line can be annexed which is incommensurable in square with the whole straight line and which with the whole straight line makes the sum of the squares on them medial, but twice the rectangle contained by them rational.
To a straight line which produces with a medial area a medial whole only one straight line can be annexed which is incommensurable in square with the whole straight line and which with the whole straight line makes the sum of the squares on them medial and twice the rectangle contained by them both medial and also incommensurable with the sum of the squares on them.
Given a rational straight line and an apotome, if the square on the whole be greater than the square on the annex by the square on a straight line commensurable in length with the whole, and the whole be commensurable in length with the rational straight line set out, let the apotome be called a first apotome.
But if the annex be commensurable in length with the rational straight line set out, and the square on the whole be greater than that on the annex by the square on a straight line commensurable with the whole, let the apotome be called a second apotome.
But if neither be commensurable in length with the rational straight line set out, and the square on the whole be greater than the square on the annex by the square on a straight line commensurable with the whole, let the apotome be called a third apotome.
Again, if the square on the whole be greater than the square on the annex by the square on a straight line incommensurable with the whole, then, if the whole be commensurable in length with the rational straight line set out, let the apotome be called a fourth apotome;
if the annex be so commensurable, a fifth;
and, if neither, a sixth.
To find the first apotome.
To find the second apotome.
To find the third apotome.
To find the fourth apotome.
To find the fifth apotome.
To find the sixth apotome.
If an area be contained by a rational straight line and a first apotome, the side
of the area is an apotome.
If an area be contained by a rational straight line and a second apotome, the side
of the area is a first apotome of a medial straight line.
If an area be contained by a rational straight line and a third apotome, the side
of the area is a second apotome of a medial straight line.
If an area be contained by a rational straight line and a fourth apotome, the side
of the area is minor.
If an area be contained by a rational straight line and a fifth apotome, the side
of the area is a straight line which produces with a rational area a medial whole.
If an area be contained by a rational straight line and a sixth apotome, the side
of the area is a straight line which produces with a medial area a medial whole.
The square on an apotome applied to a rational straight line produces as breadth a first apotome.
The square on a first apotome of a medial straight line applied to a rational straight line produces as breadth a second apotome.
The square on a second apotome of a medial straight line applied to a rational straight line produces as breadth a third apotome.
The square on a minor straight line applied to a rational straight line produces as breadth a fourth apotome.
The square on the straight line which produces with a rational area a medial whole, if applied to a rational straight line, produces as breadth a fifth apotome.
The square on the straight line which produces with a medial area a medial whole, if applied to a rational straight line, produces as breadth a sixth apotome.
A straight line commensurable in length with an apotome is an apotome and the same in order.
A straight line commensurable with an apotome of a medial straight line is an apotome of a medial straight line and the same in order.
A straight line commensurable with a minor straight line is minor.
A straight line commensurable with that which produces with a rational area a medial whole is a straight line which produces with a rational area a medial whole.
A straight line commensurable with that which produces with a medial area a medial whole is itself also a straight line which produces with a medial area a medial whole.
If from a rational area a medial area be subtracted, the side
of the remaining area becomes one of two irrational straight lines, either an apotome or a minor straight line.
If from a medial area a rational area be subtracted, there arise two other irrational straight lines, either a first apotome of a medial straight line or a straight line which produces with a rational area a medial whole.
If from a medial area there be subtracted a medial area incommensurable with the whole, the two remaining irrational straight lines arise, either a second apotome of a medial straight line or a straight line which produces with a medial area a medial whole.
The apotome is not the same with the binomial straight line.
The square on a rational straight line applied to the binomial straight line produces as breadth an apotome the terms of which are commensurable with the terms of the binomial and moreover in the same ratio; and further the apotome so arising will have the same order as the binomial straight line.
The square on a rational straight line, if applied to an apotome, produces as, breadth the binomial straight line the terms of which are commensurable with the terms of the apotome and in the same ratio; and further the binomial so arising has the same order as the apotome.
If an area be contained by an apotome and the binomial straight line the terms of which are commensurable with the terms of the apotome and in the same ratio, the side
of the area is rational.
From a medial straight line there arise irrational straight lines infinite in number, and none of them is the same as any of the preceding.
Theory of planes, coplanar lines, and solid angles
A solid is that which has length, breadth, and depth.
An extremity of a solid is a surface.
A straight line is at right angles to a plane, when it makes right angles with all the straight lines which meet it and are in the plane.
A plane is at right angles to a plane when the straight lines drawn, in one of the planes, at right angles to the common section of the planes are at right angles to the remaining plane.
The inclination of a straight line to a plane is, assuming a perpendicular drawn from the extremity of the straight line which is elevated above the plane to the plane, and a straight line joined from the point thus arising to the extremity of the straight line which is in the plane, the angle contained by the straight line so drawn and the straight line standing up.
The inclination of a plane to a plane is the acute angle contained by the straight lines drawn at right angles to the common section at the same point, one in each of the planes.
A plane is said to be similarly inclined to a plane as another is to another when the said angles of the inclinations are equal to one another.
Parallel planes are those which do not meet.
Similar solid figures are those contained by similar planes equal in multitude.
Equal and similar solid figures are those contained by similar planes equal in multitude and in magnitude.
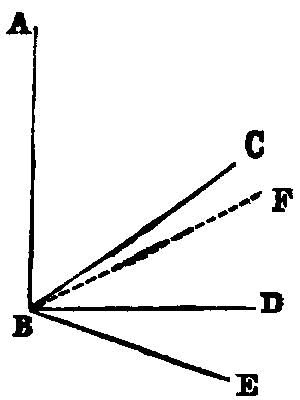
A solid angle is the inclination constituted by more than two lines which meet one another and are not in the same surface, towards all the lines.
A pyramid is a solid figure, contained by planes, which is constructed from one plane to one point.
A prism is a solid figure contained by planes two of which, namely those which are opposite, are equal, similar and parallel, while the rest are parallelograms.
When, the diameter of a semicircle remaining fixed, the semicircle is carried round and restored again to the same position from which it began to be moved, the figure so comprehended is a sphere.
The axis of the sphere is the straight line which remains fixed and about which the semicircle is turned.
The centre of the sphere is the same as that of the semicircle.
A diameter of the sphere is any straight line drawn through the centre and terminated in both directions by the surface of the sphere.
When, one side of those about the right angle in a right-angled triangle remaining fixed, the triangle is carried round and restored again to the same position from which it began to be moved, the figure so comprehended is a cone.
The axis of the cone is the straight line which remains fixed and about which the triangle is turned.
And the base is the circle described by the straight line which is carried round.
When, one side of those about the right angle in a rectangular parallelogram remaining fixed, the parallelogram is carried round and restored again to the same position from which it began to be moved, the figure so comprehended is a cylinder.
The axis of the cylinder is the straight line which remains fixed and about which the parallelogram is turned.
And the bases are the circles described by the two sides opposite to one another which are carried round.
Similar cones and cylinders are those in which the axes and the diameters of the bases are proportional.
A cube is a solid figure contained by six equal squares.
An octahedron is a solid figure contained by eight equal and equilateral triangles.
An icosahedron is a solid figure contained by twenty equal and equilateral triangles.
A dodecahedron is a solid figure contained by twelve equal, equilateral, and equiangular pentagons.

A part of a straight line cannot be in the plane of reference and a part in a plane more elevated.

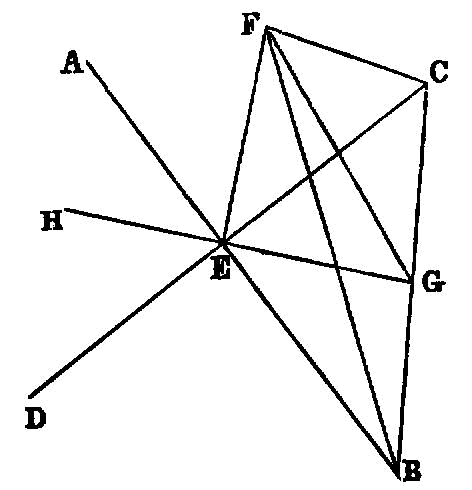
If two straight lines cut one another, they are in one plane, and every triangle is in one plane.

If two planes cut one another, their common section is a straight line.
If a straight line be set up at right angles to two straight lines which cut one another, at their common point of section, it will also be at right angles to the plane through them.
If a straight line be set up at right angles to three straight lines which meet one another, at their common point of section, the three straight lines are in one plane.
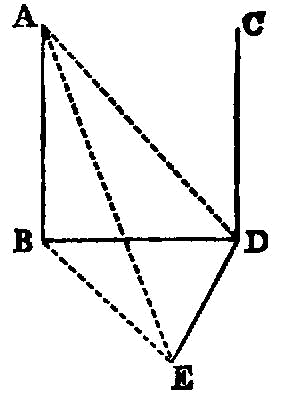
If two straight lines be at right angles to the same plane, the straight lines will be parallel.

If two straight lines be parallel and points be taken at random on each of them, the straight line joining the points is in the same plane with the parallel straight lines.
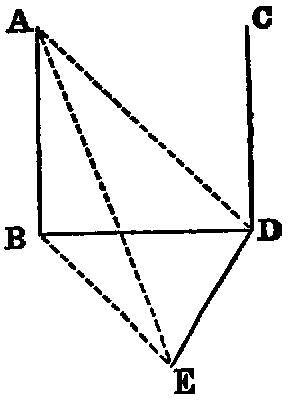
If two straight lines be parallel, and one of them be at right angles to any plane, the remaining one will also be at right angles to the same plane.
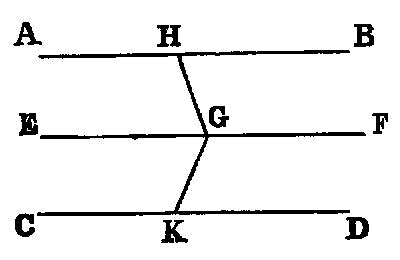
Straight lines which are parallel to the same straight line and are not in the same plane with it are also parallel to one another.
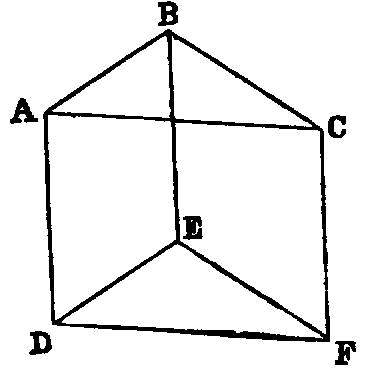
If two straight lines meeting one another be parallel to two straight lines meeting one another not in the same plane, they will contain equal angles.
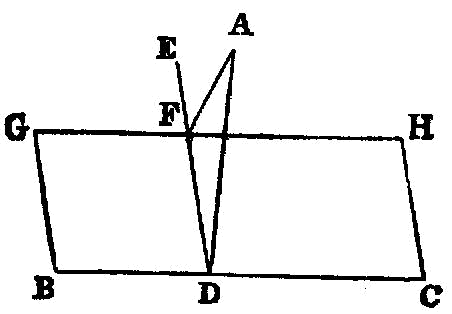
From a given elevated point to draw a straight line perpendicular to a given plane.
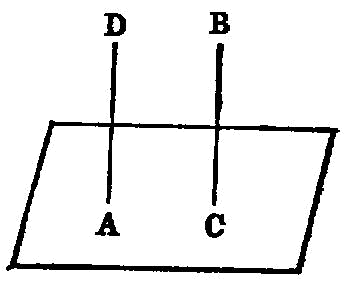
To set up a straight line at right angles to a given plane from a given point in it.
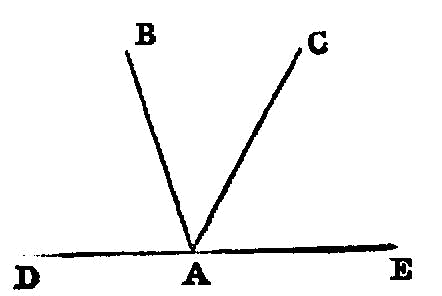
From the same point two straight lines cannot be set up at right angles to the same plane on the same side.

Planes to which the same straight line is at right angles will be parallel.

If two straight lines meeting one another be parallel to two straight lines meeting one another, not being in the same plane, the planes through them are parallel.

If two parallel planes be cut by any plane, their common sections are parallel.
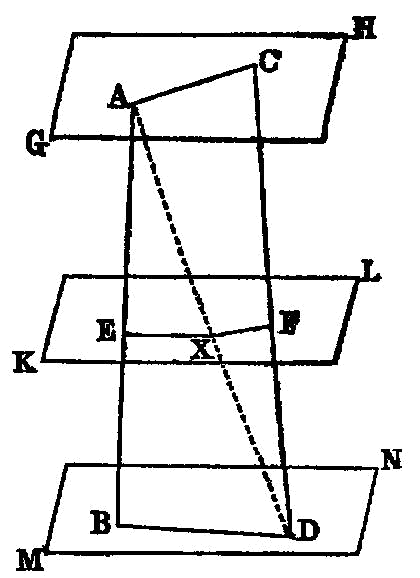
If two straight lines be cut by parallel planes, they will be cut in the same ratios.
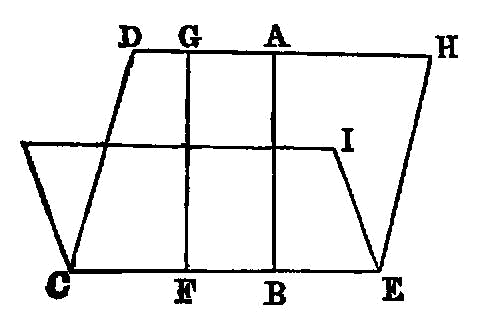
If a straight line be at right angles to any plane, all the planes through it will also be at right angles to the same plane.
If two planes which cut one another be at right angles to any plane, their common section will also be at right angles to the same plane.

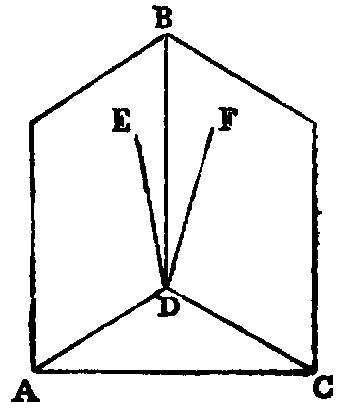
If a solid angle be contained by three plane angles, any two, taken together in any manner, are greater than the remaining one.
Any solid angle is contained by plane angles less than four right angles.
If there be three plane angles of which two, taken together in any manner, are greater than the remaining one, and they are contained by equal straight lines, it is possible to construct a triangle out of the straight lines joining the extremities of the equal straight lines.
To construct a solid angle out of three plane angles two of which, taken together in any manner, are greater than the remaining one: thus the three angles must be less than four right angles.
If a solid be contained by parallel planes, the opposite planes in it are equal and parallelogrammic.
If a parallelepipedal solid be cut by a plane which is parallel to the opposite planes, then, as the base is to the base, so will the solid be to the solid.
On a given straight line, and at a given point on it, to construct a solid angle equal to a given solid angle.
On a given straight line to describe a parallelepipedal solid similar and similarly situated to a given parallelepipedal solid.
If a parallelepipedal solid be cut by a plane through the diagonals of the opposite planes, the solid will be bisected by the plane.
Parallelepipedal solids which are on the same base and of the same height, and in which the extremities of the sides which stand up are on the same straight lines, are equal to one another.
Parallelepipedal solids which are on the same base and of the same height, and in which the extremities of the sides which stand up are not on the same straight lines, are equal to one another.
Parallelepipedal solids which are on equal bases and of the same height are equal to one another.
Parallelepipedal solids which are of the same height are to one another as their bases.
Similar parallelepipedal solids are to one another in the triplicate ratio of their corresponding sides.
In equal parallelepipedal solids the bases are reciprocally proportional to the heights; and those parallelepipedal solids in which the bases are reciprocally proportional to the heights are equal.
If there be two equal plane angles, and on their vertices there be set up elevated straight lines containing equal angles with the original straight lines respectively, if on the elevated straight lines points be taken at random and perpendiculars be drawn from them to the planes in which the original angles are, and if from the points so arising in the planes straight lines be joined to the vertices of the original angles, they will contain, with the elevated straight lines, equal angles.
If three straight lines be proportional, the parallelepipedal solid formed out of the three is equal to the parallelepipedal solid on the mean which is equilateral, but equiangular with the aforesaid solid.
If four straight lines be proportional, the parallelepipedal solids on them which are similar and similarly described will also be proportional; and, if the parallelepipedal solids on them which are similar and similarly described be proportional, the straight lines will themselves also be proportional.
If the sides of the opposite planes of a cube be bisected, and planes be carried through the points of section, the common section of the planes and the diameter of the cube bisect one another.
If there be two prisms of equal height, and one have a parallelogram as base and the other a triangle, and if the parallelogram be double of the triangle, the prisms will be equal.
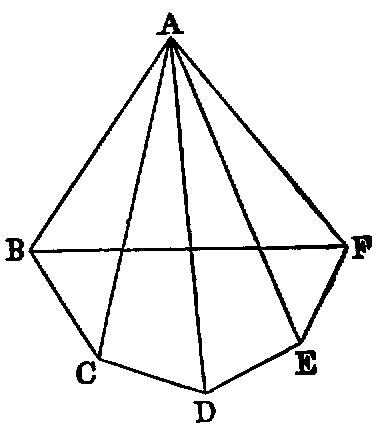
Similar polygons inscribed in circles are to one another as the squares on the diameters.
Circles are to one another as the squares on the diameters.
Any pyramid which has a triangular base is divided into two pyramids equal and similar to one another, similar to the whole and having triangular bases, and into two equal prisms; and the two prisms are greater than the half of the whole pyramid.
If there be two pyramids of the same height which have triangular bases, and cach of them be divided into two pyramids equal to one another and similar to the whole, and into two equal prisms, then, as the base of the one pyramid is to the base of the other pyramid, so will all the prisms in the one pyramid be to all the prisms, being equal in multitude, in the other pyramid.
Pyramids which are of the same height and have triangular bases are to one another as the bases.
Pyramids which are of the same height and have polygonal bases are to one another as the bases.
Any prism which has a triangular base is divided into three pyramids equal to one another which have triangular bases.
Similar pyramids which have triangular bases are in the triplicate ratio of their corresponding sides.
In equal pyramids which have triangular bases the bases are reciprocally proportional to the heights; and those pyramids in which the bases are reciprocally proportional to the heights are equal.
Any cone is a third part of the cylinder which has the same base with it and equal height.
Cones and cylinders which are of the same height are to one another as their bases.
Similar cones and cylinders are to one another in the triplicate ratio of the diameters in their bases.
If a cylinder be cut by a plane which is parallel to its opposite planes, then, as the cylinder is to the cylinder, so will the axis be to the axis.
Cones and cylinders which are on equal bases are to one another as their heights.
In equal cones and cylinders the bases are reciprocally proportional to the heights; and those cones and cylinders in which the bases are reciprocally proportional to the heights are equal.
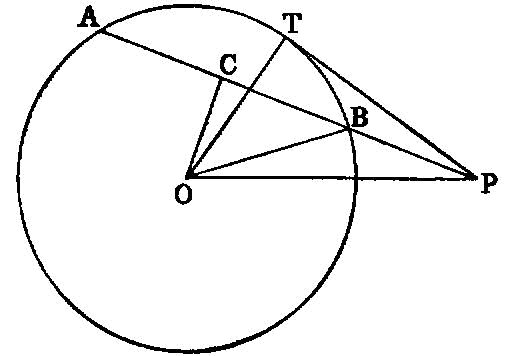
Given two circles about the same centre, to inscribe in the greater circle an equilateral polygon with an even number of sides which does not touch the lesser circle.
Given two spheres about the same centre, to inscribe in the greater sphere a polyhedral solid which does not touch the lesser sphere at its surface.
Spheres are to one another in the triplicate ratio of their respective diameters.
If a straight line be cut in extreme and mean ratio, the square on the greater segment added to the half of the whole is five times the square on the half.
If the square on a straight line be five times the square on a segment of it, then, when the double of the said segment is cut in extreme and mean ratio, the greater segment is the remaining part of the original straight line.
If a straight line be cut in extreme and mean ratio, the square on the lesser segment added to the half of the greater segment is five times the square on the half of the greater segment.
If a straight line be cut in extreme and mean ratio, the square on the whole and the square on the lesser segment together are triple of the square on the greater segment.
If a straight line be cut in extreme and mean ratio, and there be added to it a straight line equal to the greater segment, the whole straight line has been cut in extreme and mean ratio, and the original straight line is the greater segment.
If three angles of an equilateral pentagon, taken either in order or not in order, be equal, the pentagon will be equiangular.
If in an equilateral and equiangular pentagon straight lines subtend two angles taken in order, they cut one another in extreme and mean ratio, and their greater segments are equal to the side of the pentagon.
If the side of the hexagon and that of the decagon inscribed in the same circle be added together, the whole straight line has been cut in extreme and mean ratio, and its greater segment is the side of the hexagon.
If an equilateral pentagon be inscribed in a circle, the square on the side of the pentagon is equal to the squares on the side of the hexagon and on that of the decagon inscribed in the same circle.
If in a circle which has its diameter rational an equilateral pentagon be inscribed, the side of the pentagon is the irrational straight line called minor.
If an equilateral triangle be inscribed in a circle, the square on the side of the triangle is triple of the square on the radius of the circle.
To construct a pyramid, to comprehend it in a given sphere, and to prove that the square on the diameter of the sphere is one and a half times the square on the side of the pyramid.
To construct an octahedron and comprehend it in a sphere, as in the preceding case; and to prove that the square on the diameter of the sphere is double of the square on the side of the octahedron.
To construct a cube and comprehend it in a sphere, like the pyramid; and to prove that the square on the diameter of the sphere is triple of the square on the side of the cube.
To construct an icosahedron and comprehend it in a sphere, like the aforesaid figures; and to prove that the side of the icosahedron is the irrational straight line called minor.
To construct a dodecahedron and comprehend it in a sphere, like the aforesaid figures, and to prove that the side of the dodecahedron is the irrational straight line called apotome.
To set out the sides of the five figures and to compare them with one another.
assembly of research related to the project
get and stay connected with phi ARCHITECT
We are
We look forward to hearing from you
We will be in touch soon!
posting regular research and education videos
All the development code for GEOMETOR is here. As well as out content platform - PHOTON
search across entire site