Proposition I.43
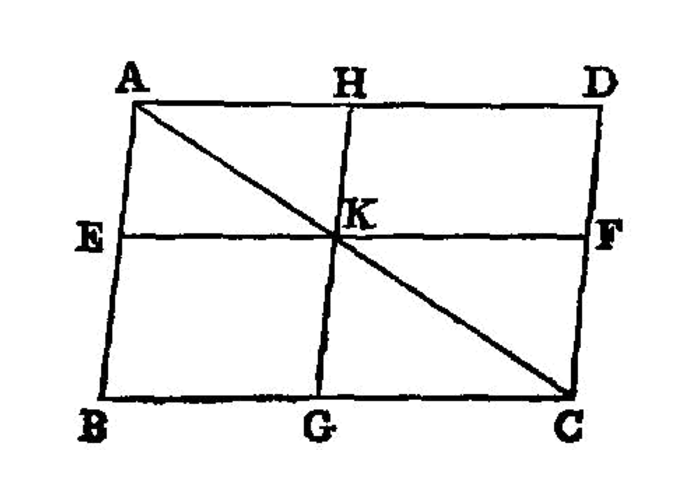
In any parallelogram the complements 1 of the parallelograms about the diameter are equal to one another.
Let ABCD be a parallelogram, and AC its diameter; and about AC let EH, FG be parallelograms, and BK, KD 2 the so-called complements;
I say that the complement BK is equal to the complement KD.
For, since ABCD is a parallelogram, and AC its diameter,
- the triangle
ABCis equal to the triangleACD. I.34
Again, since EH is a parallelogram, and AK is its diameter,
- the triangle
AEKis equal to the triangleAHK.
For the same reason
- the triangle
KFCis also equal toKGC.
Now, since the triangle AEK is equal to the triangle AHK,
- and
KFCtoKGC,
the triangle AEK together with KGC is equal to the triangle AHK together with KFC. I.c.n.2
And the whole triangle ABC is also equal to the whole ADC; therefore the complement BK which remains is equal to the complement KD which remains. I.c.n.3
Therefore etc.
- Q. E. D.
References
Footnotes
-
complements,
παραπληρώματα -
and about AC....
Euclid's phraseology here and in the next proposition implies that the complements as well as the other parallelograms areabout
the diagonal. The words are hereπερὶ δὲ τὴν ΑΓ παραλληλόγραμμα μὲν ἔστω τὰ ΕΘ, ΖΗ, τὰ δὲ λεγόμενα παραπληρώματα τὰ ΒΚ, ΚΔ . The expressionthe so-called complements
indicates that this technical use ofπαραπληρώματα was not new, though it might not be universally known. ↩
