Proposition I.22
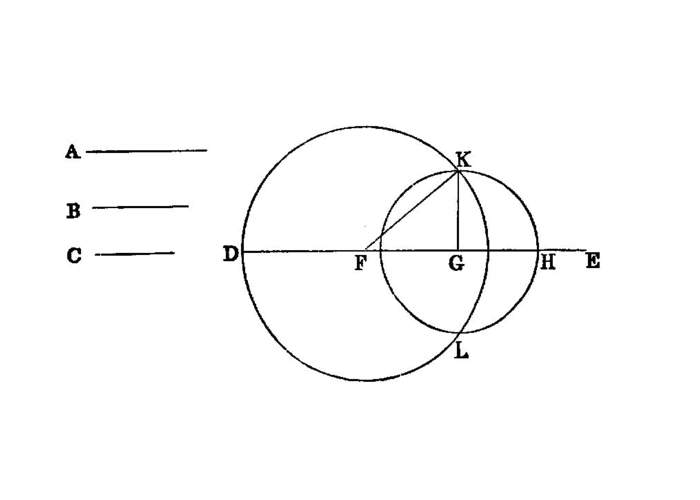
Out of three straight lines, which are equal to three given straight lines, to construct a triangle: thus it is necessary 1 that two of the straight lines taken together in any manner should be greater than the remaining one. [I.20] 2 3
Let the three given straight lines be A, B, C, and of these let two taken together in any manner be greater than the remaining one, namely A, B greater than C,
A,Cgreater thanB,
nd B, C greater than A; thus it is required to construct a triangle out of straight lines equal to A, B, C.
Let there be set out a straight line DE, terminated at D but of infinite length in the direction of E, and let DF be made equal to A, FG equal to B, and GH equal to C. I.3
With centre F and distance FD let the circle DKL be described; again, with centre G and distance GH let the circle KLH be described; and let KF, KG be joined;
I say that the triangle KFG has been constructed out of three straight lines equal to A, B, C.
For, since the point F is the centre of the circle DKL,
FDis equal toFK.
But FD is equal to A;
- therefore
KFis also equal toA.
Again, since the point G is the centre of the circle LKH,
GHis equal toGK.
But GH is equal to C;
- therefore
KGis also equal toC. AndFGis also equal toB;
therefore the three straight lines KF, FG, GK are equal to the three straight lines A, B, C.
Therefore out of the three straight lines KF, FG, GK, which are equal to the three given straight lines A, B, C, the triangle KFG has been constructed.
- Q. E. F.
References
Footnotes
-
thus it is necessary.
This is usually translated (e.g. by Williamson and Simson)
which is however inaccurate, since the Greek is notBut it is necessary,δεῖ δέ butδεῖ δή . The words are the same as those used to introduce theδιορισμός in the other sense of thedefinition
orparticular statement
of a construction to be effected. Hence, as in the latter case we saythus it is required
(e.g. to bisect the finite straight lineAB, I. 10), we should here translatethus it is necessary.
↩ -
enunciation note
This is the first case in theElements of aδιορισμός to a problem in the sense of a statement of the conditions or limits of the possibility of a solution. The criterion is of course supplied by the preceding proposition. ↩ -
enunciation note
To this enunciation all the MSS. and Boethius add, after theδιορισμός , the wordsbecause in any triangle two sides taken together in any manner are greater than the remaining one.
But this explanation has the appearance of a gloss, and it is omitted by Proclus and Campanus. Moreover there is no corresponding addition to theδιορισμός of VI. 28. ↩
