Proposition I.15
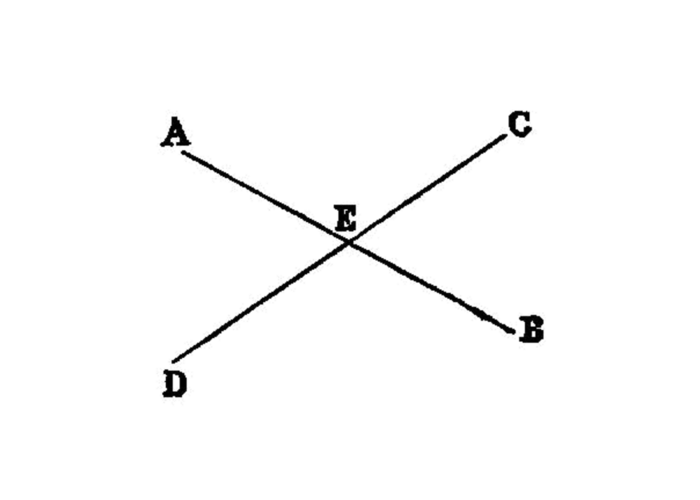
If two straight lines cut one another, they make the vertical angles 1 equal to one another.
For let the straight lines AB, CD cut one another at the point E;
I say that the angle AEC is equal to the angle DEB,
- and the angle
CEBto the angleAED.
For, since the straight line AE stands on the straight line CD, making the angles CEA, AED,
- the angles
CEA,AEDare equal to two right angles I.13
Again, since the straight line DE stands on the straight line AB, making the angles AED, DEB,
- the angles
AED,DEBare equal to two right angles. I.13
But the angles CEA, AED were also proved equal to two right angles;
- therefore the angles
CEA,AEDare equal to the anglesAED
DEB. I.post.4 and I.cn.1 Let the angleAEDbe subtracted from each; therefore the remaining angleCEAis equal to the remaining angleBED. I.cn.3
Similarly it can be proved that the angles CEB, DEA are also equal.
Therefore etc.
- Q. E. D.
Porism
[From this it is manifest that, if two straight lines cut one another, they will make the angles at the point of section 2 equal to four right angles.
References
Footnotes
-
the vertical angles.
The difference between adjacent angles (αἱ ἐφεξῆς γωνίαι ) and vertical angles (αἱ κατὰ κορυφὴν γωνίαι ) is thus explained by Proclus (p. 298, 14-24 ). The first term describes the angles made by two straight lines when one only is divded by the other, i.e. when one straight line meets another at a point which is not either of its extremities, but is not itself produced beyond the point of meeting. When the first straight line is produced, so that the lines cross at the point, they make two pairs of vertical angles (which are more clearly described as vertically opposite angles), and which are so called because their convergence is from opposite directions to one point (the intersection of the lines) as vertex (κορυφή ). ↩ -
at the point of section,
literallyat the section,
πρὸς τῇ τομῆ . ↩
