Proposition 3.15
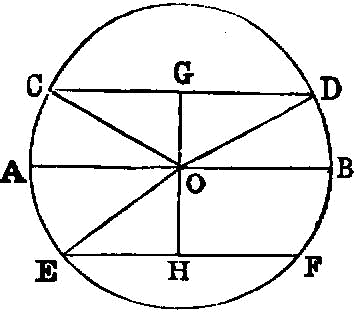
Of straight lines in a circle the diameter is greatest, and of the rest the nearer to the centre is always greater than the more remote.
Let ABCD be a circle, let AD be its diameter and E the centre; and let BC be nearer to the diameter AD, and FG more remote; I say that AD is greatest and BC greater than FG.
For from the centre E let EH, EK be drawn perpendicular to BC, FG.
Then, since BC is nearer to the centre and FG more remote, EK is greater than EH. [III. Def. 5]
Let EL be made equal to EH, through L let LM be drawn at right angles to EK and carried through to N, and let ME, EN, FE, EG be joined.
Then, since EH is equal to EL, BC is also equal to MN. [III. 14]
Again, since AE is equal to EM, and ED to EN, AD is equal to ME, EN.
But ME, EN are greater than MN, [I. 20] and MN is equal to BC; therefore AD is greater than BC.
And, since the two sides ME, EN are equal to the two sides FE, EG, and the angle MEN greater than the angle FEG, therefore the base MN is greater than the base FG. [I. 24]
But MN was proved equal to BC.
Therefore the diameter AD is greatest and BC greater than FG.
Therefore etc. Q. E. D.
