Proposition 6.2
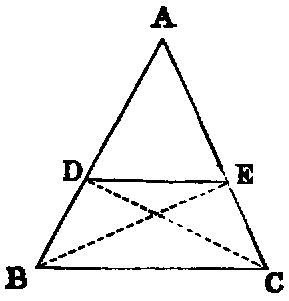
If a straight line be drawn parallel to one of the sides of a triangle, it will cut the sides of the triangle proportionally; and, if the sides of the triangle be cut proportionally, the line joining the points of section will be parallel to the remaining side of the triangle.
For let DE be drawn parallel to BC, one of the sides of the triangle ABC; I say that, as BD is to DA, so is CE to EA.
For let BE, CD be joined.
Therefore the triangle BDE is equal to the triangle CDE; for they are on the same base DE and in the same parallels DE, BC. [I. 38]
And the triangle ADE is another area.
But equals have the same ratio to the same; [V. 7] therefore, as the triangle BDE is to the triangle ADE, so is the triangle CDE to the triangle ADE.
But, as the triangle BDE is to ADE, so is BD to DA; for, being under the same height, the perpendicular drawn from E to AB, they are to one another as their bases. [VI. 1]
For the same reason also, as the triangle CDE is to ADE, so is CE to EA.
Therefore also, as BD is to DA, so is CE to EA. [V. 11]
Again, let the sides AB, AC of the triangle ABC be cut proportionally, so that, as BD is to DA, so is CE to EA; and let DE be joined.
I say that DE is parallel to BC.
For, with the same construction, since, as BD is to DA, so is CE to EA, but, as BD is to DA, so is the triangle BDE to the triangle ADE, and, as CE is to EA, so is the triangle CDE to the triangle ADE, [VI. 1] therefore also, as the triangle BDE is to the triangle ADE, so is the triangle CDE to the triangle ADE. [V. 11]
Therefore each of the triangles BDE, CDE has the same ratio to ADE.
Therefore the triangle BDE is equal to the triangle CDE; [V. 9] and they are on the same base DE.
But equal triangles which are on the same base are also in the same parallels. [I. 39]
Therefore DE is parallel to BC.
Therefore etc. Q. E. D.
